Lindelöf hypothesis
|
Read other articles:

Kazamaura-mura 風間浦村 La mairie de Kazamaura. Drapeau Administration Pays Japon Région Tōhoku Préfecture Aomori Code postal 039-4500 Démographie Population 2 550 hab. (30 avril 2014) Densité 37 hab./km2 Géographie Coordonnées 41° 29′ 15″ nord, 140° 59′ 45″ est Superficie 6 960 ha = 69,60 km2 Localisation Géolocalisation sur la carte : Japon Kazamaura-mura Géolocalisation sur la carte : Japon …

Provinsi Iwami (石見国code: ja is deprecated , iwami no kuni) adalah provinsi lama Jepang yang berada di wilayah yang sekarang menjadi bagian barat prefektur Shimane. Iwami berbatasan dengan provinsi Aki, Bingo, Izumo, Nagato, dan provinsi Suo. Ibu kota berada di kota yang sekarang disebut Hamada. Di zaman Sengoku, wilayah Iwami dikuasai klan Mōri yang berkedudukan di provinsi tetangga Aki. lbsProvinsi lama Jepang Aki Awa (Kanto) Awa (Shikoku) Awaji Bingo Bitchu Bizen Bungo Buzen Chikugo Chi…

After Met YouPoster filmSutradaraPatrick EffendyProduserRaffi AhmadRoni PariniSkenarioPatrick EffendyHaqi AhmadCeritaAri IrhamDwitasariBerdasarkanAfter Met You karya Ari Irham dan DwitasariPemeranAri IrhamYoriko AngelineNaufan Raid AzkaYudha KelingWulan GuritnoSurya SaputraPenata musikHaris PranowoDimas WibisanaSinematograferFerry RusliPenyuntingDinda AmandaPerusahaanproduksiRA PicturesTanggal rilis 10 Januari 2019 (2019-01-10) Durasi107 menitNegaraIndonesiaBahasaBahasa IndonesiaPenda…

Keuskupan Agung JakartaArchidioecesis GiakartanaKatolik Gereja Katedral JakartaLambang Keuskupan Agung JakartaLokasiNegaraIndonesiaWilayahDaerah Khusus Ibukota Jakarta (kecuali Kelurahan Pondok Labu) Tangerang Raya, Banten Kabupaten Tangerang Kota Tangerang Kota Tangerang Selatan Bekasi Raya, Jawa Barat Kabupaten Bekasi Kota Bekasi 67Provinsi gerejawiJakartaSufraganBandungBogorDekanatJakarta PusatJakarta Barat IJakarta Barat IIJakarta SelatanJakarta UtaraJakarta TimurTangerang ITangerang II…

Agence pour l'enseignement français à l'étrangerRéseau AEFE des lycées français du monde : établissements scolaires en gestion directe établissements scolaires conventionnés établissements scolaires partenaires Excellence, partage, rayonnementHistoireFondation 6 juillet 1990[1]CadreSigle AEFEZone d'activité MondeType Établissement public à caractère administratif (EPA)Forme juridique Établissement public national à caractère administratifDomaine d'activité Éducation, Fran…

العلاقات الإثيوبية البليزية إثيوبيا بليز إثيوبيا بليز تعديل مصدري - تعديل العلاقات الإثيوبية البليزية هي العلاقات الثنائية التي تجمع بين إثيوبيا وبليز.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المقارنة إثيوبي�…

JIN 「Boy With Luv」のミュージックビデオ撮影時のJIN(2019年3月撮影)基本情報原語名 진出生名 金碩珍(キム・ソクジン、김석진) 生誕 (1992-12-04) 1992年12月4日(31歳) 大韓民国 京畿道安養市 出身地 大韓民国 京畿道果川市学歴 建国大学校 映画芸術学部ジャンル K-POP職業 歌手シンガーソングライター担当楽器 ボーカル活動期間 2013年 -レーベル HYBE事務所 BIGHIT MUSIC…

Chemical compound AlaproclateClinical dataRoutes ofadministrationOralATC codeN06AB07 (WHO) Legal statusLegal status In general: uncontrolled Identifiers IUPAC name 1-(4-Chlorophenyl)-2-methylpropan-2-yl 2-aminopropanoate CAS Number60719-82-6 N 60719-83-7 (hydrochloride)PubChem CID2081ChemSpider1997 YUNIIC4R42570ZOKEGGD02787 YChEMBLChEMBL36591 YCompTox Dashboard (EPA)DTXSID5045122 Chemical and physical dataFormulaC13H18ClNO2Molar mass255.74 g·mol−13D mod…

People who live in tunnels underground This article is about homeless people. For the film, see The Mole People (film). For the science fiction characters, see Mole people (fiction). For other uses, see Mole Men (disambiguation). The examples and perspective in this article deal primarily with the United States and do not represent a worldwide view of the subject. You may improve this article, discuss the issue on the talk page, or create a new article, as appropriate. (July 2018) (Learn how and…
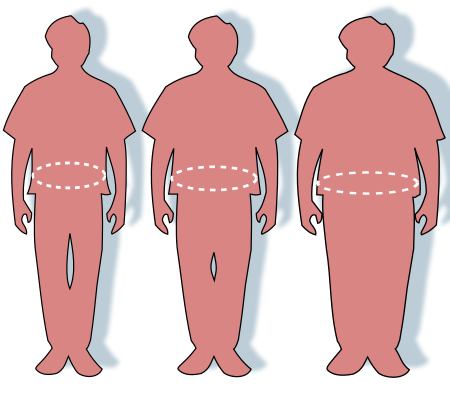
Cet article concerne l'humain. Pour le cheval, voir Masse corporelle du cheval. Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article traite essentiellement des aspects humains alors que le sujet est plus large. Il s’agit d’anthropocentrisme (janvier 2023). Discutez-en ou améliorez-le ! Pour de plus amples informations, voir le Guide contre l'anthropocentrisme. Cet article est une ébauche concernant la médecine. Vous pouvez partager vos conn…

Disambiguazione – Se stai cercando altri significati, vedi Trento (disambigua). Trentocomune Trento – VedutaTrento visione d'insieme LocalizzazioneStato Italia Regione Trentino-Alto Adige Provincia Trento AmministrazioneSindacoFranco Ianeselli (indipendente di centro-sinistra) dal 23-9-2020 TerritorioCoordinate46°04′N 11°07′E / 46.066667°N 11.116667°E46.066667; 11.116667 (Trento)Coordinate: 46°04′N 11°07′E / 46.066667�…

Об экономическом термине см. Первородный грех (экономика). ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Ранне…

CzechoslovakiaMost pointsRobert Reichel (40)IIHF codeTCHFirst international Czechoslovakia 6 - 4 Sweden (Leningrad, Soviet Union; December 27, 1973)Last international Czechoslovakia 7 - 4 Canada (Gävle, Sweden; January 4, 1993)Biggest win Czechoslovakia 21- 4 Austria (Füssen, Germany; December 28, 1980)Biggest defeat Soviet Union 9 - 1 Czechoslovakia (Karlstad, Sweden; December 30, 1978)IIHF World U20 ChampionshipAppearances20 (first in 1974)Best res…

Coordinate: 44°55′23.2″N 8°37′16.7″E / 44.923111°N 8.621306°E44.923111; 8.621306 Campo degli OrtiIl pollaioLa fabbrica del fango Cartolina che raffigura il campo e la tribuna principale (1924 circa). Informazioni generaliStato Italia Ubicazioneattuale via Milite Ignoto Inizio lavori1919 Inaugurazione19 ottobre 1919 Chiusura1929 ProprietarioAlessandria U.S. Informazioni tecnicheStrutturaPianta rettangolare CoperturaTribuna centrale Mat. del terrenotappeto erboso D…

John Stones Stones con l'Everton nel 2015 Nazionalità Inghilterra Altezza 188 cm Peso 70 kg Calcio Ruolo Difensore e Centrocampista Squadra Manchester City Carriera Giovanili 2001-2012 Barnsley Squadre di club1 2012-2013 Barnsley24 (0)2013-2016 Everton77 (1)2016- Manchester City159 (8) Nazionale 2012-2013 Inghilterra U-193 (0)2013 Inghilterra U-202 (0)2013-2015 Inghilterra U-2112 (0)2014- Inghilterra71 (3) UEFA Nations League Bronzo Portogallo 2019 Eu…

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Птиц�…

American National Standards InstituteLogo dari American National Standards Institute.SingkatanANSITanggal pendirian19 Oktober 1918; 105 tahun lalu (1918-10-19)[1]Status501(c)(3) swastaTipeOrganisasi nirlabaTujuanStandar nasionalKantor pusatWashington, D.C., U.S.Jumlah anggota 125,000 perusahaan dan 3.5 juta profesional[2]Bahasa resmi InggrisPresiden dan CEOJoe BhatiaSitus webwww.ansi.org American National Standards Institute (ANSI /ˈænsi/ AN-see) adalah sebuah lembaga nirl…

International Radiation Protection AssociationAbbreviationIRPAFormation19 June 1965Region GlobalPresidentBernard le GuenVice-PresidentChristopher ClementWebsitewww.irpa.net The International Radiation Protection Association (IRPA) is an independent non-profit association of national and regional radiation protection societies, and its mission is to advance radiation protection throughout the world. It is the international professional association for radiation protection.[1] IRPA is reco…

Aubérivecomune Aubérive – Veduta LocalizzazioneStato Francia RegioneGrand Est Dipartimento Marna ArrondissementReims CantoneMourmelon-Vesle et Monts de Champagne TerritorioCoordinate49°12′18″N 4°25′02″E / 49.205°N 4.417222°E49.205; 4.417222 (Aubérive)Coordinate: 49°12′18″N 4°25′02″E / 49.205°N 4.417222°E49.205; 4.417222 (Aubérive) Altitudine113 m s.l.m. Superficie26,17 km² Abitanti208[1] (2009) De…

Ancient coin in Greece Silver statersAn early Archaic silver stater from Corinth, 555–515 BC. Obverse: Pegasus flying left, koppa below. Reverse: quadripartite incuseSilver stater from Delphi, 338/6–334/3 BC. Obverse: head of Demeter left, wearing grain-ear wreath and veil. Reverse: Apollo seated left on omphalos, tripod to left, ΑΜΦΙΚΤΙΟΝΩΝ around. The stater (/ˈsteɪtər, stɑːˈtɛər/;[1] Ancient Greek: στατήρ, pronounced [statɛ̌ːr], romani…

























