Função identidade

Gráfico da função de identidade nos números reais.

Na matemática, uma função identidade (ou função de identidade), também chamada de relação de identidade ou mapa de identidade ou transformação de identidade, é uma função que sempre retorna o mesmo valor usado como argumento. Nas equações, a função é dada por $f(x)=x$ . Trata-se de uma função bijetiva.^[1]

Definição

Formalmente, se $X$ é um conjunto, a função de identidade $f$ em $X$ é definida para ser a função com domínio e contradomínio $X$ definida por:

${\begin{matrix}f:&X&\rightarrow &X\\&x&\mapsto &x\end{matrix}}$

ou seja, $f(x)=x$ para todos os elementos $x$ em $X$ .^[2]

Em outras palavras, o valor da função $f(x)$ em $X$ (isto é, o contradomínio) é sempre o mesmo elemento de entrada $x$ de $X$ (agora considerado como o domínio). A função de identidade em $X$ é claramente uma função injetiva, bem como uma função sobrejetiva, por isso também é bijetiva.^[3]

A função de identidade $f$ em $X$ é frequentemente denotada por $\mathrm {id} _{X}$ .

Na teoria dos conjuntos, onde uma função é definida como um tipo particular de relação binária, a função identidade é dada pela relação de identidade, ou diagonal de $X$ .

O gráfico da função identidade é uma reta bissetriz do primeiro e terceiro quadrante (x=y), ou seja, a reta passa pela origem (0,0). Por essa mesma razão ele se parece com a função linear.

Propriedade algébrica

Se $f:A\rightarrow B$ é uma função qualquer, então nós temos $f\circ \mathrm {id} _{A}=f=\mathrm {id} _{B}\circ f$ (onde " $\circ$ " denota composição de função). Em particular $\mathrm {id} _{A}$ é o elemento de identidade do monoide de todas as funções de $A$ até $A$ .

Como o elemento de identidade de um monoide é único, pode-se definir alternadamente a função de identidade $A$ ser esse elemento de identidade. Tal definição generaliza para o conceito de um morfismo de identidade na teoria de categorias, onde os endomorfismos de $A$ não deve ser funções.

Propriedades

A função identidade é um operador linear, quando aplicado a espaços vetoriais.^[4]
A função identidade nos inteiros positivos é uma função completamente multiplicativa (essencialmente multiplicação por 1), considerada na teoria dos números.^[5]
Em um espaço vetorial $n$ -dimensional, a função identidade é representada pela matriz identidade $I_{n}$ , independentemente da base.^[6]
Em um espaço métrico, a identidade é trivialmente uma isometria. Um objeto sem qualquer simetria tem como grupo de simetria o grupo trivial contendo apenas essa isometria (tipo de simetria $C_{1}$ ).^[7]
Em um espaço topológico, a função identidade é sempre contínua.

Ver também

função inclusão (por abuso de linguagem, por vezes também se chama identidade à função inclusão)

Referências

↑ Grupo de Matemática da Universidade Técnica de Lisboa. Funções. Página 10. Disponível em: <http://preprint.math.ist.utl.pt/files/ppgmutlfuncoes.pdf>. Acesso em: 07 jan 2011.
↑ Knapp, Anthony W. (2006), Basic algebra, ISBN 978-0-8176-3248-9, Springer
↑ Mapa, Sadhan Kumar. Higher Algebra Abstract and Linear 11th ed. [S.l.]: Sarat Book House. p. 36. ISBN 978-93-80663-24-1
↑ Anton, Howard (2005), Elementary Linear Algebra (Applications Version) 9th ed. , Wiley International
↑ D. Marshall; E. Odell; M. Starbird (2007). Number Theory through Inquiry. Col: Mathematical Association of America Textbooks. [S.l.]: Mathematical Assn of Amer. ISBN 978-0883857519
↑ T. S. Shores (2007). Applied Linear Algebra and Matrix Analysis. Col: Undergraduate Texts in Mathematics. [S.l.]: Springer. ISBN 038-733-195-6
↑ James W. Anderson, Hyperbolic Geometry, Springer 2005, ISBN 1-85233-934-9

[1] Grupo de Matemática da Universidade Técnica de Lisboa. Funções. Página 10. Disponível em: <http://preprint.math.ist.utl.pt/files/ppgmutlfuncoes.pdf>. Acesso em: 07 jan 2011.

[2] Knapp, Anthony W. (2006), Basic algebra, ISBN 978-0-8176-3248-9, Springer

[3] Mapa, Sadhan Kumar. Higher Algebra Abstract and Linear 11th ed. [S.l.]: Sarat Book House. p. 36. ISBN 978-93-80663-24-1

[4] Anton, Howard (2005), Elementary Linear Algebra (Applications Version) 9th ed. , Wiley International

[5] D. Marshall; E. Odell; M. Starbird (2007). Number Theory through Inquiry. Col: Mathematical Association of America Textbooks. [S.l.]: Mathematical Assn of Amer. ISBN 978-0883857519

[6] T. S. Shores (2007). Applied Linear Algebra and Matrix Analysis. Col: Undergraduate Texts in Mathematics. [S.l.]: Springer. ISBN 038-733-195-6

[7] James W. Anderson, Hyperbolic Geometry, Springer 2005, ISBN 1-85233-934-9

[1]

[2]

[3]

[4]

[5]

[6]

[7]

Agama	Bahasa	Biografi	Budaya	Ekonomi	Elektronika
Film	Filsafat	Geografi	Indonesia	Ilmu	Lingkungan
Masyarakat	Matematika	Militer	Mitologi	Musik	Olahraga
Pendidikan	Politik	Sastra	Sejarah	Seni	Teknologi

Função identidade

Definição

Propriedade algébrica

Propriedades

Ver também

Referências

Read other articles:

Radio

Imperatore del Giappone

Senam pada Pekan Olahraga Nasional XIX – Artistik serba bisa beregu putra

Associazione Sportiva Sorrento 1971-1972

Edwin Howard Armstrong

65 nm process

Electoral district of Wahroonga

Greek Dark Ages

Edinburgh

Kenner Army Health Clinic

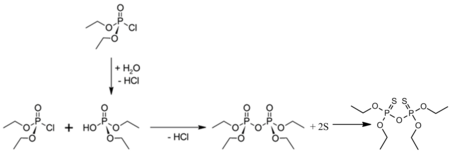
Sulfotep

Entrevue de Plombières

Шумилинская

Council of Fashion Designers of America

Konsulat Jenderal Australia, Surabaya

Філософія

Dragon Raja

Konstantinos Karamanlis

ليساندر

Waramanga, Australian Capital Territory

Portal di Ensiklopedia Dunia