Anel (matemática)
|
Read other articles:

Minka Kelly Minka Dumont Kelly (lahir 24 Juni 1980) merupakan seorang aktris berkebangsaan Amerika Serikat. Dia menjadi terkenal saat bermain di film utamanya seperti The Roommate. Dilahirkan di Los Angeles. Dia berkarier di dunia film sejak tahun 2004. Filmografi List of film credits Tahun Film Sebagai Catatan 2002 Something Corporate - If You C Jordan (Video Musik) uncredited 2004 Turbo-Charged Prelude uncredited 2005 Devil's Highway River 2006 The Pumpkin Karver Tammy Boyles State's Evidence …

Line Up Anggota 1st Generation: Aki Takajo, Ayana Shahab, Beby Chaesara Anadila, Cindy Gulla, Delima Rizky, Devi Kinal Putri, Diasta Priswarini, Frieska Anastasia Laksani, Gabriela Margareth Warouw, Ghaida Farisya, Haruka Nakagawa, Jessica Vania Widjaja, Jessica Veranda Hardja, Melody Nurramdhani Laksani, Nabilah Ratna Ayu Azalia, Rena Nozawa, Rezky Wiranti Dhike, Rica Leyona, Sendy Ariani, Shania Junianatha, Sonia Natalia, Sonya Pandarwaman, Stella Cornelia 2nd Generation: Annisa Athia, Alicia …

Jimmy Durmaz Jimmy Durmaz dari Malmö FF, 2011Informasi pribadiNama lengkap Jimmy DurmazTanggal lahir 22 Maret 1989 (umur 34)Tempat lahir Örebro, SwediaTinggi 180 cm (5 ft 11 in)Posisi bermain GelandangInformasi klubKlub saat ini ToulouseNomor 21Karier senior*Tahun Tim Tampil (Gol)2016 – Toulouse 47 (5)Tim nasional2011 – Swedia 46 (3) * Penampilan dan gol di klub senior hanya dihitung dari liga domestik Jimmy Durmaz (lahir 22 Maret 1989) adalah seorang pemain sepak bola …

Japanese light novel series and its franchise Kaze no StigmaCover of the first light novel volume (Kindle edition)風の聖痕(Kaze no Sutiguma)GenreAction, romance, supernatural Light novelWritten byTakahiro YamatoIllustrated byHanamaru NantoPublished byFujimi ShoboImprintFujimi Fantasia BunkoDemographicMaleOriginal runJanuary 18, 2002 – March 20, 2010Volumes12 MangaKaze no Stigma -Kouen no Miko-Written byTakahiro YamatoIllustrated byNeko MiyakaiPublished byFujim…

2010 single by The VaccinesWreckin' Bar (Ra Ra Ra) / Blow It UpSingle by The Vaccinesfrom the album What Did You Expect from the Vaccines? Released22 November 2010Recorded2010GenrePost-punk revival, surf punk, indie rock, garage rockLength1:24LabelMarshall TellerSongwriter(s)The VaccinesProducer(s)Dan Grech-MargueratThe Vaccines singles chronology Wreckin' Bar (Ra Ra Ra) / Blow It Up (2010) Post Break-Up Sex (2011) Wreckin' Bar (Ra Ra Ra) / Blow It Up is the debut single by English indie rock ba…

JambalayaJambalaya dengan ayam, sosis, beras, tomat, seledri, dan rempah-rempah.JenisStewBahan utamaDaging, sayuran, kaldu, nasiSunting kotak info • L • BBantuan penggunaan templat ini Media: Jambalaya Video ini menunjukkan perbedaan antara Creole dan Cajun Jambalaya. Jambalaya dibuat berbeda tergantung pada daerahnya dan terinspirasi oleh budaya Prancis dan Spanyol. Jambalaya (/[invalid input: 'icon']ˌdʒʌmbəˈlaɪ.ə/ JUM-bə-LY-ə) adalah makanan yang berasal dari L…

Bendera Brunei Darussalam Pemakaian Bendera sipil dan negara; bendera kapal sipil Perbandingan 1:2 Dipakai 29 September 1959 Rancangan Bidang kuning yang dibelah dua garis yang berwarna putih dan hitam secara diagonal; serta lambang Brunei di tengahnya. Perancang Yura Halim Bendera Brunei diperkenalkan pada 29 September 1959 semasa Brunei merupakan protektorat Britania Raya dan ditetapkan saat Brunei mencapai kemerdekaan pada 1 Januari 1984. Bendera ini terdiri dari lambang negara di bagian teng…

2001 2008 Élections cantonales françaises de 2004 21 mars 2004(1er tour)28 mars 2004(2d tour) Type d’élection Cantonales Corps électoral et résultats Inscrits au 1er tour 20 012 615 Votants au 1er tour 12 790 670 63,91 % 1,6 Votes exprimés au 1er tour 12 265 160 Blancs et nuls au 1er tour 525 510 Inscrits au 2d tour 16 620 036 Votants au 2d tour 11 048 621 66,48 % 10,2 Votes exprimés au 2…

Halaman ini berisi artikel tentang takhayul. Untuk seri televisi, lihat Jumat ke-13 (seri televisi). Artikel ini mungkin mengandung riset asli. Anda dapat membantu memperbaikinya dengan memastikan pernyataan yang dibuat dan menambahkan referensi. Pernyataan yang berpangku pada riset asli harus dihapus. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Jumat tanggal 13 ditandai di kalender Jumat-13 (atau Jumat ke-13) adalah hari yang dianggap sial dalam banyak kepercayaan takhay…

Short story by Arthur C. Clarke Critical MassShort story by Arthur C. ClarkeCountryUnited KingdomLanguageEnglishGenre(s)Science fictionPublicationPublished in LilliputPublisherPocket PublicationsPublication dateMarch 1949ChronologySeriesTales from the White Hart Armaments Race The Ultimate Melody Critical Mass is a science fiction short story by British writer Arthur C. Clarke, first published in 1949.[1] This comic story describes the relationship between a village and…

IsetnofretPermaisuri MesirIstri Kerajaan AgungDaftar Atas: Raja Ramses II, Isetnofret dan Khaemwaset di hadapan KhnumPemakamanThebes ?PasanganRamses IIAnakRamsesBintanathKhaemwasetMerenptahIsetnofret ?nama Mesir Agamaagama Mesir Kuno Isetnofret (atau Isis-nofret atau Isitnofret) (Bahasa Mesir: Isis yang cantik) merupakan salah satu Istri Kerajaan Agung Firaun Ramses II dan ibunda pewarisnya, Merneptah. Ia adalah salah satu istri kerajaan yang paling menonjol, bersama dengan N…

President of Afghanistan from 2014 to 2021 (born 1949) Ashraf Ghaniاشرف غنيGhani in 20185th President of AfghanistanIn office29 September 2014 – 15 August 2021Vice PresidentFirst VP:Abdul Rashid DostumAmrullah SalehSecond VP:Sarwar DanishChief ExecutiveAbdullah Abdullah (2014–2020)Preceded byHamid KarzaiSucceeded byHibatullah Akhundzada (as Supreme Leader)Chancellor of Kabul UniversityIn office22 December 2004 – 21 December 2008Preceded byHabibullah HabibSuccee…

Genus of extinct fishes DunkleosteusTemporal range: Late Devonian (Frasnian to Famennian), 382–358 Ma PreꞒ Ꞓ O S D C P T J K Pg N Partially reconstructed D. terrelli skull and trunk armor (specimen CMNH 5768), Cleveland Museum of Natural History Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: †Placodermi Order: †Arthrodira Suborder: †Brachythoraci Family: †Dunkleosteidae Genus: †DunkleosteusLehman, 1956 Type species †Dinichthys terrell…

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、蘭&…

Inscription made in a runic alphabet Younger futhark inscription on bone. A runic inscription is an inscription made in one of the various runic alphabets. They generally contained practical information or memorials instead of magic or mythic stories.[1] The body of runic inscriptions falls into the three categories of Elder Futhark (some 350 items, dating to between the 2nd and 8th centuries AD), Anglo-Frisian Futhorc (some 100 items, 5th to 11th centuries) and Younger Futhark (close to…

2019 single by NF When I Grow UpSingle by NFfrom the album The Search ReleasedJune 27, 2019GenreHip hopLength3:16LabelNF Real MusicCarolineSongwriter(s)Nate FeuersteinTommee ProfittProducer(s)FeuersteinProfittNF singles chronology The Search (2019) When I Grow Up (2019) Time (2019) Music videoWhen I Grow Up on YouTube When I Grow Up is a song by American rapper NF, released on June 27, 2019 as the third single from his fourth studio album The Search. Written and produced by NF and Tommee Profitt…

2017 World Weightlifting ChampionshipsMenWomen56 kg48 kg62 kg53 kg69 kg58 kg77 kg63 kg85 kg69 kg94 kg75 kg105 kg90 kg+105 kg+90 kgvte Main article: 2017 World Weightlifting Championships The Men's 56 kg competition at the 2017 World Weightlifting Championships was held on 29 November 2017.[1][2][3] Schedule Date Time Event 29 November 2017 13:55 Group A Medalists Event Gold Silver Bronze Snatch Thạch Kim Tuấn (VIE) 126 kg Trần Lê Quốc Toàn …

Species of moth Comostola laesaria Mounted specimen Living specimen Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Arthropoda Class: Insecta Order: Lepidoptera Family: Geometridae Genus: Comostola Species: C. laesaria Binomial name Comostola laesaria(Walker, 1861) Synonyms Iodis laesaria Walker, 1861 Eucrostis perlepidaria Walker, 1866 Comostola laesaria is a moth of the family Geometridae first described by Francis Walker in 1861. It is found in Sri Lanka, India, Ind…

Hari Menanam Pohon di Korea. Hansik (한식; 寒食) adalah salah satu dari perayaan besar di Korea, selain hari Seollal(설날), Dano(단오) dan Chuseok(추석). Pada hari Hansik, orang-orang tidak menyalakan api untuk memasak atau menghangatkan makanan. Hari Hansik berakar dari cerita dan ritual kuno Tionghoa yang pertama kali diperingati pada masa Periode Musim Semi dan Musim Gugur saat Chong-er, seorang bangsawan Wen dari negara Jin (晋) secara tidak sengaja membunuh bawahan dan teman baik…
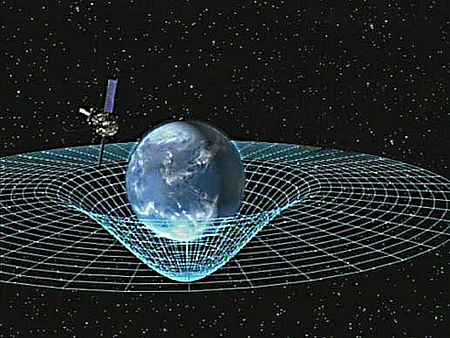
Spacetime used in theory of relativity For the fictional concept in the Gundam franchise, see Gundam Universal Century technology § Minovsky physics.For the use in algebraic number theory, see Minkowski space (number field). This article needs editing to comply with Wikipedia's Manual of Style. In particular, it has problems with MOS:BBB for ℝ. Please help improve the content. (June 2024) (Learn how and when to remove this message) Hermann Minkowski (1864–1909) found that the theory of…












































































