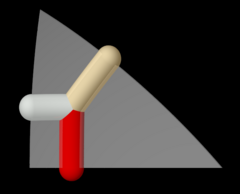
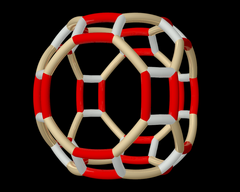
Wythoff construction
|
Read other articles:

ProDG (pronounced “prodigy”) by SN Systems is a suite of development tools produced for PlayStation 3, PlayStation 2, PSP, Nintendo DS, GameCube, and Game Boy Advance. The phrase PRO-DG was originally registered as a UK trademark,[1] but the hyphen was never used for a released version and the suite has subsequently been known as ProDG. The suite consisted of console hardware-specific assemblers, a C/C++ compiler, ELF/DLL linkers, and a source-level debugger. The build tools could be…

Lillian RichRich c. 1924Lahir(1900-01-01)1 Januari 1900Herne Hill, London, EnglandMeninggal5 Januari 1954(1954-01-05) (umur 54)Woodland Hills, Los Angeles, California, A.S.PekerjaanAktrisTahun aktif1919–1940 Lillian Rich (1 Januari 1900 – 5 Januari 1954) adalah seorang aktris kelahiran Inggris dari era bisu. Dia muncul di 66 film antara tahun 1919 dan 1940.[1][2] Referensi ^ Lillian Rich. tcm.com. Diakses tanggal 11 September 2018. ^ Goldsborough…

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (يوليو 2019) منتخب إندونيسيا تحت 20 سنة لكرة القدم بلد الرياضة إندونيسيا الفئة كرة قدم تحت 20 سنة للرجال [لغات أخر…

For the villages in Iran, see Danian, Iran. First age of the Paleocene Epoch Danian66.0 – 61.6 Ma PreꞒ Ꞓ O S D C P T J K Pg N ↓ Chronology−65 —–−60 —–−55 —–−50 —–−45 —–−40 —–−35 —–−30 —–−25 —–MZCenozoicKPaleogeneNLKPaleoceneEoceneOligoceneMCMaastricht.DanianSelandianThanetianYpres…

FOMO (akronim dari fear of missing out), takut ketinggalan kereta, takut ketinggalan bus, atau takut kudet merupakan perasaan cemas yang timbul karena sesuatu yang menarik dan menyenangkan sedang terjadi, sering disebabkan karena unggahan di media sosial.[1] FOMO didefinisikan sebagai rasa takut karena tertinggal atau tidak mengetahui peristiwa, informasi, atau pengalaman, dan orang lain mendapat pengalaman berharga dari sesuatu tersebut. Ditandai adanya keinginan untuk terus terhubung d…

Warna hijau pada lampu lalu-lintas berarti jalan. Lampu hijau merupakan isyarat atau izin untuk menjalankan suatu rencana/proyek.[1] Istilah ini mengacu pada sinyal hijau lampu lalu-lintas, yang berarti jalan. Dalam konteks industri film dan televisi, memberikan lampu hijau berarti menyetujui secara resmi dana produksi dan berkomitmen atas pembiayaan tersebut, sehingga memungkinkan proyek untuk bergerak maju dari tahap pengembangan menuju pra-produksi dan pengambilan gambar utama. Orang-…

Poci dengan motif hiasan bunga. Poci atau teko (Inggris: teapotcode: en is deprecated ) adalah suatu wadah yang digunakan untuk menjerang daun teh atau campuran herbal dengan air yang hampir mendidih. Teh dapat ditempatkan dalam kantung teh celup atau dibiarkan tersebar. Jika dibiarkan tersebar, diperlukan saringan teh untuk menangkap daun-daun teh di dalam poci sewaktu akan menuang. Poci biasanya memiliki tutup di bagian atasnya untuk tempat memasukkan teh dan air, gagang untuk memegangnya, ser…

Insiden TapaniOrang Taiwan yang ditawan setelah Insiden Tapani dibawa dari penjara Tainan ke pengadilanTanggal1915LokasiTaiwanHasil Kemenangan JepangPihak terlibat Orang Han TaiwanPenduduk asli Taiwan Kekaisaran JepangTokoh dan pemimpin Yu Qingfang Kekuatan 1.413[1][2] Insiden Tapani Nama Tionghoa Hanzi: 噍吧哖事件 Alih aksara Mandarin - Hanyu Pinyin: Jiaòbānián Shìjìan - Wade-Giles: Chiao4-pa1-nien2 Shih4-chien4 Min Nan - Romanisasi POJ: Ta-pa-nî sū-kiāⁿ Alt…

Balai kota Peta Slowakia yang menunjukkan Ružomberok Ružomberok (bahasa Jerman: Rosenberg, bahasa Hungaria: Rózsahegy) adalah sebuah kota di Region Zilina, Slowakia utara. Pariwisata Skipark Ruzomberok yang memiliki jalur sepanjang 12 km, ialah salah satu dari 5 stasiun ski utama di Slowakia.[butuh rujukan] Stasiun ini dikenal karena memiliki kereta gantung Gondola modern yang menghubungkan kota dengan stasiun di ketinggian, begitupun dengan sebuah chairlift, juga 9 teleski. Jalu…

Iowa State Senate elections, 2014 ← 2012 November 4, 2014 2016 → 25 out of 50 seats in the Iowa State Senate26 seats needed for a majority Majority party Minority party Leader Pam Jochum Bill Dix Party Democratic Republican Leader's seat 50th district 25th district Last election 26 24 Seats before 26 24 Seats after 26 24 Seat change Results of the elections: Democratic gain Rep…

الكلية الكندية الدوليةالمعهد الكندي العالي شعار الكلية الكندية الدولية معلومات التأسيس 2004 (منذ 20 سنة) النوع معهد خاص المعاهد هندسة وإعلام وإدارة الشُعب 4 الموقع الجغرافي إحداثيات 30°02′05″N 31°25′46″E / 30.0347°N 31.4295°E / 30.0347; 31.4295 المدينة فرعين بالشيخ زايد والقاهرة �…

Bridge in Paris, France This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Pont Saint-Louis – news · newspapers · books · scholar · JSTOR (May 2019) (Learn how and when to remove this template message) Pont Saint-LouisCoordinates48°51′10″N 02°21′10″E / 48.85278°N 2.35278°E / 48.85278; 2.35278Loca…

Public college in Montréal, Quebec Collège Bois-de-BoulogneMottoVivez l'expérience (French)Typepublic collegeEstablished1967Administrative staff450Undergraduates2700 pre-university and technical students; 4400 adult education and corporate programsAddress10555, avenue de Bois-de-BoulogneMontreal, QuebecH4N 1L4CampusUrban/Suburban,Sports teamsCavaliersColoursBlue and Green AffiliationsACCC, CCAA, QSSF, AUCC,Websitewww.bdeb.qc.ca/ The Collège Bois-de-Boulogne is a French-language …

NaskahUncial 076TeksKisah Para Rasul 2WaktuAbad ke-5/ke-6Aksarabahasa YunaniKini diPierpont Morgan LibraryUkuran17 x 15 cmJenisTeks AlexandriaKategoriII Uncial 076 (dalam penomoran Gregory-Aland), α1008 (von Soden); adalah sebuah naskah kuno yang memuat sebagian Perjanjian Baru di Alkitab Kristen, khususnya Kisah Para Rasul. Ditulis dalam gaya tulisan uncial dalam bahasa Yunani, berdasarkan Paleografi diperkirakan dibuat pada abad ke-5 atau ke-6. Dahulunya diberi label יa.[1] Peme…

Cet article est une ébauche concernant une commune du Calvados. Vous pouvez partager vos connaissances en l’améliorant (comment ?). Le bandeau {{ébauche}} peut être enlevé et l’article évalué comme étant au stade « Bon début » quand il comporte assez de renseignements encyclopédiques concernant la commune. Si vous avez un doute, l’atelier de lecture du projet Communes de France est à votre disposition pour vous aider. Consultez également la page d’aide à la …
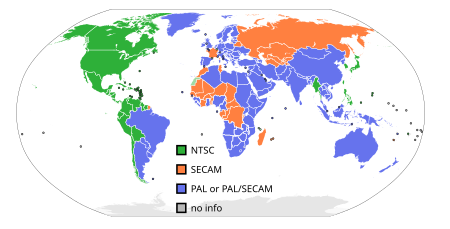
artikel ini perlu dirapikan agar memenuhi standar Wikipedia. Tidak ada alasan yang diberikan. Silakan kembangkan artikel ini semampu Anda. Merapikan artikel dapat dilakukan dengan wikifikasi atau membagi artikel ke paragraf-paragraf. Jika sudah dirapikan, silakan hapus templat ini. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Artikel ini bukan mengenai Vidio. Tiga macam sinyal video analog yang dipakai di seluruh dunia: NTSC, PAL, SECAM Video adalah suatu bentuk media visu…

Artikel ini bukan mengenai C. R. Rao. C.N.R. RaoLahir30 Juni 1934 (umur 89)Bangalore, Kerajaan Mysore, India BritaniaTempat tinggalIndiaKebangsaanIndiaAlmamaterUniversitas MysoreUniversitas Hindu BanarasPurdue UniversityDikenal atasKimia benda padatSains materialPenghargaanPenghargaan Shanti Swarup Bhatnagar untuk Ilmu Pengetahuan dan Teknologi (1969)Medali Hughes (2000)Penghargaan Sains India (2004)Medali Abdus Salam (2008)Medali Dan David (2005)Legion of Honor (2005)Royal Medal (2009)Padm…

British writer and hispanist For the Australian rules footballer, see Gerald Brennan (footballer). Gerald BrenanBornEdward FitzGerald Brenan(1894-04-07)7 April 1894Sliema, British Malta[1]Died19 January 1987(1987-01-19) (aged 92)Alhaurin el Grande, SpainOccupation(s)Author, historian Edward FitzGerald Gerald Brenan, CBE, MC (7 April 1894 – 19 January 1987)[2] was a British writer and hispanist who spent much of his life in Spain. Brenan is probably best known for The Spani…

U.S. botanist (1858–1954) Liberty Hyde BaileyBorn(1858-03-15)March 15, 1858South Haven, Michigan, U.S.DiedDecember 25, 1954(1954-12-25) (aged 96)Ithaca, New York, U.S.Alma materMichigan Agricultural College (now Michigan State University)AwardsVeitch Memorial Medal (1897)Scientific careerFieldsBotanyInstitutionsMichigan State University, Cornell UniversityAuthor abbrev. (botany)L.H. Bailey Liberty Hyde Bailey (March 15, 1858 – December 25, 1954) was an American horticulturist …

Brand of apparel PINKCompany typeSubsidiaryIndustryApparelFoundedOctober 16, 2002; 21 years ago (2002-10-16)HeadquartersReynoldsburg, Ohio, United StatesNumber of locations141 stores (2020)Key peopleAmy Hauk, CEO (2019)ProductsApparel, lingerieParentVictoria's SecretWebsitevictoriassecret.com/pink Pink (stylized PINK) is a lingerie and apparel line by Victoria's Secret, a former subsidiary of L Brands, targeting younger women than their main line. The target demographic skews y…