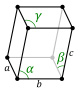
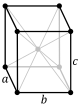
晶系
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

American physicist This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Stephen Bernard Libby – news · newspapers · books · scholar · JSTOR (December 2019) (Learn how and when to remove this template me…

Miss Teen USALogo Miss Teen USATanggal pendirian1983TipeKontes kecantikanKantor pusatNew YorkLokasi Amerika SerikatBahasa resmi Bahasa InggrisDirektur NasionalCrystle StewartOrganisasi indukMiss USAAfiliasiMicrosoftSony Interactive EntertainmentSitus webmissteenusa.com Miss Teen USA adalah kontes kecantikan dijalankan oleh Organisasi Miss Universe untuk remaja perempuan berusia 14–19 tahun. Tidak seperti kontes saudaranya Miss Universe dan Miss USA, yang disiarkan di Fox, kontes ini disia…

1986 fantasy comedy film SuperfantagenioDirected byBruno CorbucciWritten byMario Amendola, Bruno Corbucci, Elisa BrigantiProduced byYoram GlobusMenahem GolanStarringBud SpencerLuca VenantiniJanet AgrenJulian VoloshinDistributed byCannon FilmsRelease date December 25, 1986 (1986-12-25) Running time96 minutesCountriesItalyUnited StatesLanguagesItalianEnglish Superfantagenio (also known as Aladdin) is a 1986 Italian/American family comedy film starring Bud Spencer as the Genie and Lu…

This is a list of newspapers in Rhode Island. Daily newspapers The Boston Globe (Rhode Island) of Boston, owned by Boston Globe Media Partners, via their Providence-based bureau, covering all of Rhode Island The Brown Daily Herald of Providence, owned independently, covering Brown University The Call of Woonsocket, owned by RISN Operations, covering northern Providence County Kent County Daily Times of West Warwick, owned by RISN Operations, covering most of Kent County The Newport Daily News of…

Radio station in Farmington, MissouriKTJJFarmington, MissouriBroadcast areaSte. GenevieveSt. LouisCape GirardeauFrequency98.5 MHzBrandingJ98 The BootProgrammingFormatCountry musicAffiliationsABC News RadioPremiere NetworksWestwood OneOwnershipOwnerAlpha Media(Alpha Media Licensee LLC)Sister stationsKREI, KJFFTechnical informationFacility ID35533ClassCERP100,000 wattsHAAT317 meters (1,040 ft)Transmitter coordinates37°43′7.00″N 90°33′1.00″W / 37.7186111°N 90.5502778…

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Птиц�…

City in Razavi Khorasan province, Iran For the administrative divisions, see Golmakan District and Golmakan Rural District. City in Razavi Khorasan, IranGolmakan Persian: گلمكانCityGolmakanCoordinates: 36°28′56″N 59°09′33″E / 36.48222°N 59.15917°E / 36.48222; 59.15917[1]CountryIranProvinceRazavi KhorasanCountyGolbaharDistrictGolmakanPopulation (2016)[2] • Total8,373Time zoneUTC+3:30 (IRST) Golmakan (Persian: گلمكان,…

ChodkiewiczAlternative name(s)Kościesza odm. ChodkiewiczFamilies1 names Chodkiewicz (Chodźkiewicz) DivisionsGmina Gródek, Gródek Chodkiewicz (Gryf z Mieczem) is a Polish coat of arms. It was used by the Chodkiewicz family in the times of the Polish–Lithuanian Commonwealth. A variant of the Kościesza with the Gryf coat of arms and the notable longer family line as well as much bigger family than shown here. Notable bearers Notable bearers of this coat of arms include: Chodkiewicz family Ch…

ロバート・デ・ニーロRobert De Niro 2011年のデ・ニーロ生年月日 (1943-08-17) 1943年8月17日(80歳)出生地 アメリカ合衆国・ニューヨーク州ニューヨーク市身長 177 cm職業 俳優、映画監督、映画プロデューサージャンル 映画、テレビドラマ活動期間 1963年 -配偶者 ダイアン・アボット(1976年 - 1988年)グレイス・ハイタワー(1997年 - )主な作品 『ミーン・ストリート』(1973年)『…

Group D of UEFA Euro 2012 began on 11 June 2012 and ended on 19 June 2012. The pool was made up of Ukraine, Sweden, France and England. The top two teams, England and France, progressed to the quarter-finals to play Italy and Spain respectively, while Ukraine and Sweden were eliminated from the tournament. On the second matchday, on 15 June, the match between Ukraine and France that began at 19:00 local time was interrupted by heavy rainfall and a thunderstorm. The conditions forced the referee …

Pour les articles homonymes, voir Cardinale (homonymie). Claudia Cardinale Claudia Cardinale, durant le tournage du filmLa Panthère rose (1963). Données clés Nom de naissance Claude Joséphine Rose Cardinale Surnom CC Naissance 15 avril 1938 (86 ans)Tunis (Protectorat français de Tunisie) Nationalité Italienne Profession ActriceMannequin Films notables CartoucheHuit et demiLe GuépardLa Mafia fait la loiIl était une fois dans l'Ouest Site internet official-claudiacardinale.com modifie…

Rapid transit line in Metro Vancouver, Canada Canada Line Canada Line train pulling into Marine Drive stationOverviewOwnerTransLink, InTransitBCLocaleMetro Vancouver, British ColumbiaTerminiWaterfrontYVR–Airport & Richmond–BrighouseStations16 (1 under construction)Websitethecanadaline.comServiceTypeRapid transitSystemSkyTrainOperator(s)ProTrans BCRolling stockRotem EMU, 2 cars per trainsetDaily ridership94,000 (2022)[1]HistoryOpenedAugust 17, 2009; 14 years ago …

State park in California, United States Morro Strand State BeachShow map of CaliforniaShow map of the United StatesLocationSan Luis Obispo County, CaliforniaNearest cityMorro BayCoordinates35°22′33″N 120°51′47″W / 35.37583°N 120.86306°W / 35.37583; -120.86306Governing bodyCalifornia Department of Parks and Recreation Orca breaching, just offshore from Morro Strand, May 8, 2014. North Morro Bay town in background Morro Strand State Beach (formerly Ata…

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Ни�…

Robert Kurrle Adossé à la caméra (photo dédicacée, 1916) Données clés Nom de naissance Robert Bard Kurrle Naissance 2 février 1890Port HuenemeCalifornie, États-Unis Nationalité Américaine Décès 27 octobre 1932 (à 42 ans)Los Angeles (Hollywood)Californie, États-Unis Profession Directeur de la photographie Films notables RamonaFaiblesse humaineLes Quatre Plumes blanchesVoyage sans retour modifier Sur le tournage de Ramona (1928), avec Dolores del Río et l'aviateur Charles Lipp…

Titan bulan Saturnus dalam warna alami Kolonisasi angkasa Planet Dalam Lagrange Merkurius Venus Bumi Bulan Mars Ceres Planet Luar Jupiter Europa Kalisto Saturnus Titan Uranus Objek trans-Neptunus lbs Menurut data Cassini dari 2008, Titan memiliki ratusan kali hidrokarbon cair lebih banyak daripada semua cadangan minyak dan gas alam yang diketahui di Bumi. Hidrokarbon ini berupa hujan dari langit dan terkumpul dalam endapan besar yang membentuk danau dan bukit pasir.[1] Titan hanya tertut…

Japanese weekly news magazine Shūkan ShinchōCover of the first issue of Shūkan Shinchō (19 February 1956)CategoriesNews magazineFrequencyWeeklyFounded1956First issue19 February 1956CompanyShincho-shaCountryJapanBased inTokyoLanguageJapaneseWebsiteShukan Shincho Shūkan Shinchō (週刊新潮) is a Japanese conservative[1][2] weekly news magazine based in Tokyo, Japan. It is considered one of the most influential weekly magazines in the country[2] and is the first Japa…

American politician (1853–1918) Charles W. FultonUnited States Senatorfrom OregonIn officeMarch 4, 1903 – March 3, 1909Preceded byJoseph SimonSucceeded byGeorge Earle ChamberlainPresident of the Oregon State SenateIn office1893–18941901–1902Preceded byJoseph SimonT. C. TaylorSucceeded byJoseph SimonGeorge C. BrownellOregon State SenatorIn office1878–18811891–18951898–1903ConstituencyClatsop, Columbia, and Tillamook counties Personal detailsBorn(1853-08-24)Au…

FLSW redirects here. For the airport with ICAO code FLSW, see Solwezi Airport. For the reserve unit of the U.S. Navy, see Fleet Logistics Support Wing. Learned society headquartered in Cardiff, Wales The Learned Society of WalesCymdeithas Ddysgedig CymruFormation25 May 2010; 13 years ago (25 May 2010)TypeLearned Society; National AcademyRegistration no.1168622Legal statusCharityPurposeTo advance education, learning, academic study and knowledge, so as to contribute to scientifi…

烏克蘭總理Прем'єр-міністр України烏克蘭國徽現任杰尼斯·什米加尔自2020年3月4日任命者烏克蘭總統任期總統任命首任維托爾德·福金设立1991年11月后继职位無网站www.kmu.gov.ua/control/en/(英文) 乌克兰 乌克兰政府与政治系列条目 宪法 政府 总统 弗拉基米尔·泽连斯基 總統辦公室 国家安全与国防事务委员会 总统代表(英语:Representatives of the President of Ukraine) 总理…