此條目介紹的是基本概念。关于高阶的主題,请见「
群論 」。
魔方 的所有可能重新排列形成一個群,叫做魔方群 。在數學中,群 (英語:group )是指配備二元運算 的集合 ,其二元運算需要具有結合律 、單位元 和逆元素 。因為眾多數學結構都是群(如整數系 配備上加法 就形成一個群),因而可以簡潔地從不同的數學結構歸納出共通的結果,这使群成為當代數學的核心概念。[ 1] [ 2]
很多自然界的變換(如平移 、鏡射 )的匯總都符合群的定義,而某群變換下保持不變的某種性質被稱為对称性 ;如在空間對稱群 的哪些變換下,面积 或角度 會保持不變,就是在研究立体几何 的对称性。
抽象群的現代概念是從多個數學領域發展出來的。[ 3] [ 4] [ 5] 多項式 方程 。十九世紀法國數學家埃瓦里斯特·伽罗瓦 ,擴展了保罗·鲁菲尼 和约瑟夫·拉格朗日 先前的工作,依據特定多項式方程的根 (解)的對稱群給出了對它的可解性的判别准则。這個伽罗瓦群 的元素對應於根的特定置換 。伽罗瓦的想法最初被同代人所拒絕,只在死后才出版。[ 6] [ 7] 置換群 由奥古斯丁·路易·柯西 專門研究。阿瑟·凱萊 的“On the theory of groups, as depending on the symbolic equation
ϑ
n
=
1
{\displaystyle \vartheta ^{n}=1}
有限群 的第一個抽象定義。[ 8]
幾何是第二個系统性的使用群,特別是對稱群 的領域。这类群是菲利克斯·克莱因 1872 年的爱尔兰根纲领 的一部分。[ 9] 雙曲幾何 和射影幾何 形成之后,克萊因利用群論 以更連貫的方式來組織它們。索菲斯·李 進一步發展了這些想法,在 1884 年創立了李群 的研究。[ 10]
对群論有貢獻的第三個領域是數論 。一些阿貝爾群 結構在卡爾·弗里德里希·高斯 的數論著作《算术研究 》(1798 年)中被隐含地用到,并被利奥波德·克罗内克 更明顯地用到。[ 11] 恩斯特·库默尔 發展了描述用素数 做因數分解 的理想類群 ,使證明費馬大定理 的早期嘗試達到了高潮。[ 12]
把上述各種來源融合成一个群的統一理論是从卡米尔·若尔当 的“Traité des substitutions et des équations algébriques” (1870 年)開始的。[ 13] 瓦尔特·冯·迪克 (1882年)給出了第一个抽象群的現代定義的陳述。[ 14] 费迪南德·格奥尔格·弗罗贝尼乌斯 和威廉·伯恩赛德 的开拓性著作中獲得了廣泛的认识,他們研究有限群的表示理論 ,還有理查德·布劳尔 的模表示論 和 Issai Schur 的論文。[ 15] 赫尔曼·外尔 、埃利·嘉当 和很多其他人推進了李群和更一般的局部緊群 的理論。[ 16] 代數群 的理論,由克劳德·舍瓦莱 (從 1930 年代晚期开始)和后來阿尔曼德·波莱尔 和雅克·蒂茨 的重要著作奠基。[ 17]
芝加哥大学 于 1960-61 年举办的“群论年”活动促使群论家们以丹尼尔·戈伦斯坦 ,约翰·格里格斯·汤普森 和瓦爾特·法伊特 为基础展开合作。在大量其他数学家的帮助下,他们完成了有限单群的分类 。这项工程,不论是从证明长度来说还是从参与人数来说,其浩大程度超越了之前一切的数学成果。简化此证明的研究还在进行中。[ 18] [a]
給定集合
G
{\displaystyle G}
二元運算
∘
:
G
×
G
→
G
{\displaystyle \circ :G\times G\to G}
∘
(
a
,
b
)
{\displaystyle \circ (a,\,b)}
a
∘
b
{\displaystyle a\circ b}
[ 19]
结合律
對所有
g
1
,
g
2
,
g
3
∈
G
{\displaystyle g_{1},\,g_{2},\,g_{3}\in G}
g
1
∘
(
g
2
∘
g
3
)
=
(
g
1
∘
g
2
)
∘
g
3
{\displaystyle g_{1}\circ (g_{2}\circ g_{3})=(g_{1}\circ g_{2})\circ g_{3}}
左单位元 與左逆元素
存在
e
∈
G
{\displaystyle e\in G}
g
∈
G
{\displaystyle g\in G}
e
∘
g
=
g
{\displaystyle e\circ g=g}
且存在
γ
∈
G
{\displaystyle \gamma \in G}
γ
∘
g
=
e
{\displaystyle \gamma \circ g=e}
的話,稱
(
G
,
∘
)
{\displaystyle (G,\ \circ )}
群 。當其配備的二元運算
∘
{\displaystyle \circ }
(
G
,
∘
)
{\displaystyle (G,\ \circ )}
G
{\displaystyle G}
群运算的次序很重要,也就是說,等式
a
∘
b
=
b
∘
a
{\displaystyle a\circ b=b\circ a}
交换群 (或阿貝爾群 ,以尼尔斯·阿贝尔 命名),不满足交换律的群称为非交换群(非阿贝尔群)。如以下面舉例 一節的二面體群 就不是交换群。
上面關於單位元和逆元素的部分也可以改為:
右单位元 與右逆元素
存在
e
∈
G
{\displaystyle e\in G}
g
∈
G
{\displaystyle g\in G}
g
∘
e
=
g
{\displaystyle g\circ e=g}
且存在
γ
∈
G
{\displaystyle \gamma \in G}
g
∘
γ
=
e
{\displaystyle g\circ \gamma =e}
因為不管原來的淡紫色定義,還是淡黃色的替代性定義,配上結合律 都會等價於以下的定義:
单位元 與逆元素
存在
e
∈
G
{\displaystyle e\in G}
g
∈
G
{\displaystyle g\in G}
e
∘
g
=
g
∘
e
=
g
{\displaystyle e\circ g=g\circ e=g}
且存在
γ
∈
G
{\displaystyle \gamma \in G}
γ
∘
g
=
g
∘
γ
=
e
{\displaystyle \gamma \circ g=g\circ \gamma =e}
證明
若二元運算
∘
:
G
×
G
→
G
{\displaystyle \circ :G\times G\to G}
e
l
∈
G
{\displaystyle e_{l}\in G}
g
∈
G
{\displaystyle g\in G}
「
e
l
∘
g
=
g
{\displaystyle e_{l}\circ g=g}
「存在某
γ
l
∈
G
{\displaystyle \gamma _{l}\in G}
γ
l
∘
g
=
e
l
{\displaystyle \gamma _{l}\circ g=e_{l}}
因為
∘
{\displaystyle \circ }
二元運算 ,
g
∘
γ
∈
G
{\displaystyle g\circ \gamma \in G}
Γ
∈
G
{\displaystyle \Gamma \in G}
Γ
∘
(
g
∘
γ
l
)
=
e
l
{\displaystyle \Gamma \circ (g\circ \gamma _{l})=e_{l}}
這樣根據結合律和 (left-1) 與 (left-2) 有
e
l
=
Γ
∘
(
g
∘
γ
l
)
=
Γ
∘
[
g
∘
(
e
l
∘
γ
l
)
]
=
Γ
∘
{
g
∘
[
(
γ
l
∘
g
)
∘
γ
l
]
}
=
Γ
∘
{
g
∘
[
γ
l
∘
(
g
∘
γ
l
)
]
}
=
Γ
∘
[
(
g
∘
γ
l
)
∘
(
g
∘
γ
l
)
]
=
[
Γ
∘
(
g
∘
γ
l
)
]
∘
(
g
∘
γ
l
)
=
e
l
∘
(
g
∘
γ
l
)
=
g
∘
γ
l
{\displaystyle {\begin{aligned}e_{l}&=\Gamma \circ (g\circ \gamma _{l})\\&=\Gamma \circ [\,g\circ (e_{l}\circ \gamma _{l})\,]\\&=\Gamma \circ \{\,g\circ [\,(\gamma _{l}\circ g)\circ \gamma _{l}\,]\,\}\\&=\Gamma \circ \{\,g\circ [\,\gamma _{l}\circ (g\circ \gamma _{l})\,]\,\}\\&=\Gamma \circ [\,(g\circ \gamma _{l})\circ (g\circ \gamma _{l})\,]\\&=[\,\Gamma \circ (g\circ \gamma _{l})\,]\circ (g\circ \gamma _{l})\\&=e_{l}\circ (g\circ \gamma _{l})\\&=g\circ \gamma _{l}\end{aligned}}}
所以左逆元必為右逆元 ,這樣根據結合律有
g
∘
e
l
=
g
∘
(
γ
l
∘
g
)
=
(
g
∘
γ
l
)
∘
g
=
e
l
∘
g
=
g
{\displaystyle {\begin{aligned}g\circ e_{l}&=g\circ (\gamma _{l}\circ g)\\&=(g\circ \gamma _{l})\circ g\\&=e_{l}\circ g=g\end{aligned}}}
所以左單位元必為右單位元 。
類似地,若二元運算
∘
:
G
×
G
→
G
{\displaystyle \circ :G\times G\to G}
e
r
∈
G
{\displaystyle e_{r}\in G}
g
∈
G
{\displaystyle g\in G}
「
g
∘
e
r
=
g
{\displaystyle g\circ e_{r}=g}
「存在某
γ
r
∈
G
{\displaystyle \gamma _{r}\in G}
g
∘
γ
r
=
e
r
{\displaystyle g\circ \gamma _{r}=e_{r}}
因為
γ
r
∘
g
∈
G
{\displaystyle \gamma _{r}\circ g\in G}
Γ
¯
∈
G
{\displaystyle {\overline {\Gamma }}\in G}
(
γ
r
∘
g
)
∘
Γ
¯
=
e
r
{\displaystyle (\gamma _{r}\circ g)\circ {\overline {\Gamma }}=e_{r}}
所以根據結合律、(right-1) 和 (right-2) 有
e
r
=
(
γ
r
∘
g
)
∘
Γ
¯
=
[
(
γ
r
∘
e
r
)
∘
g
]
∘
Γ
¯
=
{
[
γ
r
∘
(
g
∘
γ
r
)
]
∘
g
}
∘
Γ
¯
=
{
[
(
γ
r
∘
g
)
∘
γ
r
]
∘
g
}
∘
Γ
¯
=
[
(
γ
r
∘
g
)
∘
(
γ
r
∘
g
)
]
∘
Γ
¯
=
(
γ
r
∘
g
)
∘
[
(
γ
r
∘
g
)
∘
Γ
¯
]
=
(
γ
r
∘
g
)
∘
e
r
=
γ
r
∘
g
{\displaystyle {\begin{aligned}e_{r}&=(\gamma _{r}\circ g)\circ {\overline {\Gamma }}\\&=[\,(\gamma _{r}\circ e_{r})\circ g\,]\circ {\overline {\Gamma }}\\&=\{\,[\,\gamma _{r}\circ (g\circ \gamma _{r})\,]\circ g\,\}\circ {\overline {\Gamma }}\\&=\{\,[\,(\gamma _{r}\circ g)\circ \gamma _{r}\,]\circ g\,\}\circ {\overline {\Gamma }}\\&=[\,(\gamma _{r}\circ g)\circ (\gamma _{r}\circ g)\,]\circ {\overline {\Gamma }}\\&=(\gamma _{r}\circ g)\circ [\,(\gamma _{r}\circ g)\circ {\overline {\Gamma }}\,]\\&=(\gamma _{r}\circ g)\circ e_{r}\\&=\gamma _{r}\circ g\end{aligned}}}
所以右逆元也為左逆元 。這樣根據結合律有
e
r
∘
g
=
(
g
∘
γ
r
)
∘
g
=
g
∘
(
γ
r
∘
g
)
=
g
∘
e
r
=
g
{\displaystyle {\begin{aligned}e_{r}\circ g&=(g\circ \gamma _{r})\circ g\\&=g\circ (\gamma _{r}\circ g)\\&=g\circ e_{r}=g\end{aligned}}}
所以右單位元必為左單位元 。
再考慮到淡藍色是要求
e
∈
G
{\displaystyle e\in G}
整數系
Z
{\displaystyle \mathbb {Z} }
…
,
−
4
,
−
3
,
−
2
,
−
1
,
0
,
1
,
2
,
3
,
4
,
…
{\displaystyle \dots ,\,-4,\,-3,\,-2,\,-1,\,0,\,1,\,2,\,3,\,4,\dots }
[ 20] 可以看出,整数系和整数的加法是可以构成群的:
對于任意兩個整數
a
{\displaystyle a}
b
{\displaystyle b}
a
+
b
{\displaystyle a+b}
二元运算 ,換言之,滿足封閉性。
對于任意三個整數
a
,
b
,
c
{\displaystyle a,\,b,\,c}
(
a
+
b
)
+
c
=
a
+
(
b
+
c
)
{\displaystyle (a+b)+c=a+(b+c)}
a
{\displaystyle a}
b
{\displaystyle b}
c
{\displaystyle c}
a
{\displaystyle a}
b
{\displaystyle b}
c
{\displaystyle c}
對於任意整數
a
{\displaystyle a}
a
+
0
=
0
+
a
=
a
{\displaystyle a+0=0+a=a}
0
{\displaystyle 0}
a
{\displaystyle a}
−
a
{\displaystyle -a}
a
+
(
−
a
)
=
(
−
a
)
+
a
=
0
{\displaystyle a+(-a)=(-a)+a=0}
a
{\displaystyle a}
实数 集去掉
0
{\displaystyle 0}
R
♯
:=
R
∖
{
0
}
{\displaystyle \mathbb {R} ^{\sharp }:=\mathbb {R} \backslash \{0\}}
∀
a
,
b
∈
R
♯
,
a
b
∈
R
♯
{\displaystyle \forall a,\,b\in \mathbb {R} ^{\sharp },\,ab\in \mathbb {R} ^{\sharp }}
∀
a
,
b
,
c
∈
R
♯
,
(
a
b
)
c
=
a
(
b
c
)
{\displaystyle \forall a,\,b,\,c\in \mathbb {R} ^{\sharp },\,(ab)c=a(bc)}
對於
1
∈
R
♯
{\displaystyle 1\in \mathbb {R} ^{\sharp }}
∀
a
∈
R
♯
,
a
1
=
1
a
=
a
{\displaystyle \forall a\in \mathbb {R} ^{\sharp },\,a1=1a=a}
∀
a
∈
R
♯
,
∃
a
−
1
∈
R
♯
,
a
(
a
−
1
)
=
(
a
−
1
)
a
=
1
{\displaystyle \forall a\in \mathbb {R} ^{\sharp },\,\exists a^{-1}\in \mathbb {R} ^{\sharp },\,a\left(a^{-1}\right)=\left(a^{-1}\right)a=1}
以下是正方形的 8 个旋轉 和翻轉:
id
{\displaystyle \operatorname {id} }
r
1
{\displaystyle r_{1}}
r
2
{\displaystyle r_{2}}
r
3
{\displaystyle r_{3}}
f
v
{\displaystyle f_{v}}
f
h
{\displaystyle f_{h}}
f
d
{\displaystyle f_{d}}
f
c
{\displaystyle f_{c}}
注意顏色不同,「操作結果」才不同。數字只是去方便理解「操作過程」,數字有沒有顛倒不影響「操作結果」。
如果
a
{\displaystyle a}
b
{\displaystyle b}
a
∘
b
{\displaystyle a\circ b}
a
{\displaystyle a}
b
{\displaystyle b}
r
3
{\displaystyle r_{3}}
f
h
{\displaystyle f_{h}}
f
d
{\displaystyle f_{d}}
r
3
∘
f
h
=
f
d
{\displaystyle r_{3}\circ f_{h}=f_{d}}
下面的群表 列出了這種「操作的複合」的所有可能結果。
∘
{\displaystyle \circ }
先操作
id
{\displaystyle \operatorname {id} }
r
1
{\displaystyle r_{1}}
r
2
{\displaystyle r_{2}}
r
3
{\displaystyle r_{3}}
f
v
{\displaystyle f_{v}}
f
h
{\displaystyle f_{h}}
f
d
{\displaystyle f_{d}}
f
c
{\displaystyle f_{c}}
後操作
id
{\displaystyle \operatorname {id} }
id
{\displaystyle \operatorname {id} }
r
1
{\displaystyle r_{1}}
r
2
{\displaystyle r_{2}}
r
3
{\displaystyle r_{3}}
f
v
{\displaystyle f_{v}}
f
h
{\displaystyle f_{h}}
f
d
{\displaystyle f_{d}}
f
c
{\displaystyle f_{c}}
r
1
{\displaystyle r_{1}}
r
1
{\displaystyle r_{1}}
r
2
{\displaystyle r_{2}}
r
3
{\displaystyle r_{3}}
id
{\displaystyle \operatorname {id} }
f
c
{\displaystyle f_{c}}
f
d
{\displaystyle f_{d}}
f
v
{\displaystyle f_{v}}
f
h
{\displaystyle f_{h}}
r
2
{\displaystyle r_{2}}
r
2
{\displaystyle r_{2}}
r
3
{\displaystyle r_{3}}
id
{\displaystyle \operatorname {id} }
r
1
{\displaystyle r_{1}}
f
h
{\displaystyle f_{h}}
f
v
{\displaystyle f_{v}}
f
c
{\displaystyle f_{c}}
f
d
{\displaystyle f_{d}}
r
3
{\displaystyle r_{3}}
r
3
{\displaystyle r_{3}}
id
{\displaystyle \operatorname {id} }
r
1
{\displaystyle r_{1}}
r
2
{\displaystyle r_{2}}
f
d
{\displaystyle f_{d}}
f
c
{\displaystyle f_{c}}
f
h
{\displaystyle f_{h}}
f
v
{\displaystyle f_{v}}
f
v
{\displaystyle f_{v}}
f
v
{\displaystyle f_{v}}
f
d
{\displaystyle f_{d}}
f
h
{\displaystyle f_{h}}
f
c
{\displaystyle f_{c}}
id
{\displaystyle \operatorname {id} }
r
2
{\displaystyle r_{2}}
r
1
{\displaystyle r_{1}}
r
3
{\displaystyle r_{3}}
f
h
{\displaystyle f_{h}}
f
h
{\displaystyle f_{h}}
f
c
{\displaystyle f_{c}}
f
v
{\displaystyle f_{v}}
f
d
{\displaystyle f_{d}}
r
2
{\displaystyle r_{2}}
id
{\displaystyle \operatorname {id} }
r
3
{\displaystyle r_{3}}
r
1
{\displaystyle r_{1}}
f
d
{\displaystyle f_{d}}
f
d
{\displaystyle f_{d}}
f
h
{\displaystyle f_{h}}
f
c
{\displaystyle f_{c}}
f
v
{\displaystyle f_{v}}
r
3
{\displaystyle r_{3}}
r
1
{\displaystyle r_{1}}
id
{\displaystyle \operatorname {id} }
r
2
{\displaystyle r_{2}}
f
c
{\displaystyle f_{c}}
f
c
{\displaystyle f_{c}}
f
v
{\displaystyle f_{v}}
f
d
{\displaystyle f_{d}}
f
h
{\displaystyle f_{h}}
r
1
{\displaystyle r_{1}}
r
3
{\displaystyle r_{3}}
r
2
{\displaystyle r_{2}}
id
{\displaystyle \operatorname {id} }
如果取
D
4
=
{
id
,
r
1
,
r
2
,
r
3
,
f
v
,
f
h
,
f
d
,
f
c
}
{\displaystyle D_{4}=\left\{\operatorname {id} ,\,\,r_{1},\,r_{2},\,r_{3},\,f_{v},\,f_{h},\,f_{d},\,f_{c}\right\}}
那麼根據以上的群表,
∘
:
D
4
×
D
4
→
D
4
{\displaystyle \circ :D_{4}\times D_{4}\to D_{4}}
二元运算 ,而且
(
D
4
,
∘
)
{\displaystyle (D_{4},\,\circ )}
id
{\displaystyle \operatorname {id} }
二面體群 。注意到上表淡紫色的部分破壞了交換律,所以二面體群不是交换群 。
若群
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
e
∈
G
{\displaystyle e\in G}
e
′
∈
G
{\displaystyle e^{\prime }\in G}
g
∈
G
{\displaystyle g\in G}
g
=
e
∘
g
=
g
∘
e
{\displaystyle g=e\circ g=g\circ e}
g
=
e
′
∘
g
=
g
∘
e
′
{\displaystyle g=e^{\prime }\circ g=g\circ e^{\prime }}
這樣的話,把
g
{\displaystyle g}
e
′
{\displaystyle e^{\prime }}
e
{\displaystyle e}
e
′
=
e
∘
e
′
=
e
′
∘
e
{\displaystyle e^{\prime }=e\circ e^{\prime }=e^{\prime }\circ e}
e
=
e
′
∘
e
=
e
∘
e
′
{\displaystyle e=e^{\prime }\circ e=e\circ e^{\prime }}
所以
e
′
=
e
{\displaystyle e^{\prime }=e}
所以群的單位元是唯一的,這樣根據函數符號與唯一性間的關係 ,可以添加新的三元函數符號
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
合式公式
B
{\displaystyle {\mathcal {B}}}
∘
{\displaystyle \circ }
G
{\displaystyle G}
二元运算 ,且存在
∘
{\displaystyle \circ }
[
¬
B
∧
(
e
(
G
,
∘
)
=
∅
)
]
∨
[
B
∧
(
∀
g
∈
G
)
(
e
(
G
,
∘
)
∘
g
=
g
∘
e
(
G
,
∘
)
=
g
)
]
{\displaystyle [\neg {\mathcal {B}}\wedge (e_{(G,\,\circ )}=\varnothing )]\vee [{\mathcal {B}}\wedge (\forall g\in G)(e_{(G,\,\circ )}\circ g=g\circ e_{(G,\,\circ )}=g)]}
這條公理直觀上表示,只要「
∘
{\displaystyle \circ }
G
{\displaystyle G}
∘
{\displaystyle \circ }
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
空集 。
為了簡便起見,
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
e
G
{\displaystyle e_{G}}
e
{\displaystyle e}
在增添以上的新函數符號
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
若群
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
g
∈
G
{\displaystyle g\in G}
γ
∈
G
{\displaystyle \gamma \in G}
γ
¯
∈
G
{\displaystyle {\bar {\gamma }}\in G}
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
e
G
=
g
∘
γ
=
γ
∘
g
{\displaystyle e_{G}=g\circ \gamma =\gamma \circ g}
e
G
=
g
∘
γ
′
=
γ
′
∘
g
{\displaystyle e_{G}=g\circ \gamma ^{\prime }=\gamma ^{\prime }\circ g}
那這樣的話,依據定義裡的结合律 和
e
(
G
,
∘
)
{\displaystyle e_{(G,\,\circ )}}
γ
¯
=
γ
¯
∘
e
G
=
γ
¯
∘
(
g
∘
γ
)
=
(
γ
¯
∘
g
)
∘
γ
=
e
G
∘
γ
=
γ
{\displaystyle {\begin{aligned}{\bar {\gamma }}&={\bar {\gamma }}\circ e_{G}\\&={\bar {\gamma }}\circ (g\circ \gamma )\\&=({\bar {\gamma }}\circ g)\circ \gamma \\&=e_{G}\circ \gamma \\&=\gamma \end{aligned}}}
所以任意
g
∈
G
{\displaystyle g\in G}
函數符號與唯一性間的關係 ,可以添加一個三元函數符號
g
−
1
(
G
,
∘
)
{\displaystyle {g^{-1}}_{(G,\,\circ )}}
合式公式
D
{\displaystyle {\mathcal {D}}}
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
g
∈
G
{\displaystyle g\in G}
[
¬
D
∧
(
g
−
1
(
G
,
∘
)
=
∅
)
]
∨
[
D
∧
(
g
−
1
(
G
,
∘
)
∘
g
=
g
−
1
(
G
,
∘
)
∘
g
=
e
G
)
]
{\displaystyle [\neg {\mathcal {D}}\wedge ({g^{-1}}_{(G,\,\circ )}=\varnothing )]\vee [{\mathcal {D}}\wedge ({g^{-1}}_{(G,\,\circ )}\circ g={g^{-1}}_{(G,\,\circ )}\circ g=e_{G})]}
這條公理直觀上表示,只要「
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
g
∈
G
{\displaystyle g\in G}
g
−
1
(
G
,
∘
)
{\displaystyle {g^{-1}}_{(G,\,\circ )}}
g
{\displaystyle g}
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
上對應的那個唯一逆元素」,否則取
g
−
1
(
G
,
∘
)
{\displaystyle {g^{-1}}_{(G,\,\circ )}}
空集 。
簡便起見,
g
−
1
(
G
,
∘
)
{\displaystyle {g^{-1}}_{(G,\,\circ )}}
g
−
1
{\displaystyle g^{-1}}
若
(
G
,
∘
)
{\displaystyle \left(G,\,\circ \right)}
整数 指數 ,對任意
g
∈
G
{\displaystyle g\in G}
递归定义
g
0
:=
e
{\displaystyle g^{0}:=e}
單位元 視為
0
{\displaystyle 0}
g
1
:=
g
{\displaystyle g^{1}:=g}
對所有的整数
n
∈
Z
{\displaystyle n\in \mathbb {Z} }
g
n
+
1
:=
g
n
∘
g
{\displaystyle g^{n+1}:=g^{n}\circ g}
對所有的整数
n
∈
Z
{\displaystyle n\in \mathbb {Z} }
g
n
−
1
:=
g
n
∘
g
−
1
{\displaystyle g^{n-1}:=g^{n}\circ g^{-1}}
有時,某個群的運算可以跟直觀上的加法聯想在一起,這個運算也可以改記為「
+
{\displaystyle +}
⊕
{\displaystyle \oplus }
f
+
g
−
1
{\displaystyle f+g^{-1}}
f
−
g
{\displaystyle f-g}
f
⊖
g
{\displaystyle f\ominus g}
f
−
g
{\displaystyle f-g}
減法 。更有甚者,
g
−
1
{\displaystyle g^{-1}}
−
g
{\displaystyle -g}
類似的,如果群的運算可以跟直觀上的乘法聯想在一起而改記為「
×
{\displaystyle \times }
⊗
{\displaystyle \otimes }
f
×
g
−
1
{\displaystyle f\times g^{-1}}
f
÷
g
{\displaystyle f\div g}
f
g
{\displaystyle {\frac {f}{g}}}
除法 ;更有甚者,
g
−
1
{\displaystyle g^{-1}}
1
g
{\displaystyle {\frac {1}{g}}}
注意以上都是為了直觀理解方便所規定的簡寫 ,並不是斷定群的運算必然跟一般实数 的加減乘除一模一樣。
本章節羅列一些群論涉及到的概念,其并非群本身的固有性質,但其與群的應用息息相關。本章節使用了數學符號 如
X
=
{
x
,
y
,
z
}
{\displaystyle X=\{x,\,y,\,z\}}
集合
X
{\displaystyle X}
元素
x
,
y
{\displaystyle x,\,y}
z
{\displaystyle z}
x
∈
X
{\displaystyle x\in X}
x
{\displaystyle x}
X
{\displaystyle X}
f
:
,
X
→
Y
{\displaystyle f\colon ,\,X\to Y}
f
{\displaystyle f}
X
{\displaystyle X}
Y
{\displaystyle Y}
函數 。
要超越上述純粹符號操作水平去理解群,必須采用更加結構性的概念。[c] 集合 就沒有)的优势,與群有關的構造必須与群運算兼容 。下列概念中以各種方式表现了這種兼容性。例如,群可以通過叫做群同態的函數 相互關聯。根據上述這個原理,要求它們以精確的意义照顧到群結構。群的結構還可以通過把它們分解成子群和商群來理解。“保持結構”的原理是在數學中反復出現的一個主題,它是靠範疇 來工作的一個實例,在這裡的情況下靠群范疇 。[ 21]
群同态[g]
φ
:
G
→
H
{\displaystyle \varphi \colon \,G\to H}
φ
(
a
∘
G
b
)
=
φ
(
a
)
∘
H
φ
(
b
)
{\displaystyle \varphi (a\circ _{G}b)=\varphi (a)\circ _{H}\varphi (b)}
對
G
{\displaystyle G}
a
,
b
{\displaystyle a,\,b}
φ
(
e
G
)
=
e
H
{\displaystyle \varphi (e_{G})=e_{H}}
∀
a
∈
G
,
φ
(
a
−
1
)
=
[
φ
(
a
)
]
−
1
{\displaystyle \forall a\in G,\,\varphi (a^{-1})=\left[\varphi (a)\right]^{-1}}
[ 22]
兩群
G
,
H
{\displaystyle G,\,H}
同構 的,當且僅當存在群同態
ρ
:
G
→
H
,
σ
:
H
→
G
{\displaystyle \rho \colon \,G\to H,\,\sigma \colon \,H\to G}
ρ
σ
=
id
G
,
σ
ρ
=
id
H
{\displaystyle \rho \sigma =\operatorname {id} _{G},\,\sigma \rho =\operatorname {id} _{H}}
G
{\displaystyle G}
H
{\displaystyle H}
恆等變換 ,此時也記
σ
=
φ
−
1
{\displaystyle \sigma =\varphi ^{-1}}
G
{\displaystyle G}
a
{\displaystyle a}
a
a
=
e
G
{\displaystyle aa=e_{G}}
H
{\displaystyle H}
φ
{\displaystyle \varphi }
φ
(
a
)
φ
(
a
)
=
φ
(
e
G
)
=
e
H
{\displaystyle \varphi (a)\varphi (a)=\varphi (e_{G})=e_{H}}
此外,一個群可以擁有非恆等變換的自同態 或自同构 ,如果以映射為元,映射复合 為群乘法,則任意群
G
{\displaystyle G}
End
G
{\displaystyle \operatorname {End} G}
Aut
G
{\displaystyle \operatorname {Aut} G}
非正式地說,子群是包含在更大的群内的一個群。[ 23]
具體而論,對群
G
{\displaystyle G}
H
⊆
G
{\displaystyle H\subseteq G}
H
{\displaystyle H}
G
{\displaystyle G}
∘
G
{\displaystyle \circ _{G}}
e
G
∈
H
{\displaystyle e_{G}\in H}
H
{\displaystyle H}
G
{\displaystyle G}
H
≤
G
{\displaystyle H\leq G}
可以證明,可通過以下判據校驗群
G
{\displaystyle G}
H
{\displaystyle H}
H
≤
G
⇔
∀
g
,
h
∈
H
,
g
−
1
h
∈
H
{\displaystyle H\leq G\Leftrightarrow \forall g,\,h\in H,\,g^{-1}h\in H}
前文所述的例子 中,幺元與旋轉構成其一個子群
R
=
{
id
,
r
1
,
r
2
,
r
3
}
{\displaystyle R=\left\{\operatorname {id} ,\,r_{1},\,r_{2},\,r_{3}\right\}}
了解子群族 對于作為一個整體來理解群是重要的。[d]
給定群
G
{\displaystyle G}
S
⊆
G
{\displaystyle S\subseteq G}
S
{\displaystyle S}
⟨
S
⟩
=
{
e
,
s
1
s
2
⋯
s
n
:
∀
i
,
s
i
∈
S
∪
S
−
1
}
{\displaystyle \langle S\rangle =\left\{e,s_{1}s_{2}\cdots s_{n}\colon \,\forall i,\,s_{i}\in S\cup S^{-1}\right\}}
S
−
1
:=
{
s
−
1
:
s
∈
S
}
{\displaystyle S^{-1}:=\left\{s^{-1}\colon \,s\in S\right\}}
S
{\displaystyle S}
G
{\displaystyle G}
[ 24]
S
{\displaystyle S}
有限 乘積組成。
同樣在前文所述的二面體群中存在例子:由
{
r
2
,
f
v
}
{\displaystyle \left\{r_{2},\,f_{v}\right\}}
{
id
,
r
2
,
f
v
,
f
h
=
f
v
r
2
}
{\displaystyle \left\{\operatorname {id} ,\,r_{2},\,f_{v},\,f_{h}=f_{v}r_{2}\right\}}
∀
a
,
a
2
=
id
{\displaystyle \forall a,a^{2}=\operatorname {id} }
階 。
在很多情況下,需要認為兩個元是等同的,如果它們只相差一個给定子群中的元素。例如,在上述
D
4
{\displaystyle D_{4}}
r
2
{\displaystyle r_{2}}
H
{\displaystyle H}
H
{\displaystyle H}
g
{\displaystyle g}
H
{\displaystyle H}
g
{\displaystyle g}
G
/
H
=
{
x
H
:
x
∈
G
}
{\displaystyle G/H=\left\{xH\colon \,x\in G\right\}}
H
∖
G
=
{
H
x
:
x
∈
G
}
{\displaystyle H\backslash G=\left\{Hx\colon \,x\in G\right\}}
其中
左陪集
g
H
=
{
g
h
:
h
∈
H
}
{\displaystyle gH=\left\{gh\colon \,h\in H\right\}}
H
g
=
{
h
g
:
h
∈
H
}
{\displaystyle Hg=\left\{hg\colon \,h\in H\right\}}
[ 25] 同時也可以定義雙陪集為
H
∖
G
/
K
=
{
H
x
K
:
x
∈
G
,
H
x
K
=
{
h
x
k
:
h
∈
H
,
k
∈
K
}
}
{\displaystyle H\backslash G/K=\left\{HxK\colon \,x\in G,\,HxK=\left\{hxk\colon \,h\in H,\,k\in K\right\}\right\}}
顯然,左右陪集為雙陪集之特例,取某一側子群為
{
e
}
{\displaystyle \{e\}}
任何子群
H
{\displaystyle H}
G
{\displaystyle G}
劃分 ,換言之,
對任兩個陪集
H
x
K
,
H
y
K
{\displaystyle HxK,\,HyK}
H
x
K
∩
H
y
K
≠
∅
⇔
H
x
K
=
H
y
K
{\displaystyle HxK\cap HyK\neq \varnothing \Leftrightarrow HxK=HyK}
取陪集所有元作無交并 有
G
=
⨆
x
H
x
K
{\displaystyle G=\bigsqcup _{x}HxK}
x
{\displaystyle x}
H
{\displaystyle H}
G
{\displaystyle G}
g
{\displaystyle g}
g
H
=
H
g
{\displaystyle gH=Hg}
H
{\displaystyle H}
正規子群 。
在前面介绍的對稱群
D
4
{\displaystyle D_{4}}
R
{\displaystyle R}
g
R
{\displaystyle gR}
要么等于
R
{\displaystyle R}
g
∈
R
{\displaystyle g\in R}
要么等于
U
=
f
v
R
=
{
f
v
,
f
d
,
f
h
,
f
c
}
{\displaystyle U=f_{v}R=\left\{f_{v},\,f_{d},\,f_{h},\,f_{c}\right\}}
此處的子群
R
{\displaystyle R}
D
4
{\displaystyle D_{4}}
f
v
R
=
U
=
R
f
v
{\displaystyle f_{v}R=U=Rf_{v}}
f
v
{\displaystyle f_{v}}
有时,在由陪集形成的集合上可以赋予一个满足群公理的运算,使之成为商群 或因子群 。这仅在子群正规时可行。給定任何對
G
{\displaystyle G}
N
{\displaystyle N}
N
{\displaystyle N}
(
G
/
N
,
∘
)
{\displaystyle (G/N,\,\circ )}
∘
:
(
x
N
,
y
N
)
↦
x
y
N
{\displaystyle \circ \colon \,(xN,\,yN)\mapsto xyN}
這個定義是由關聯任何元素
g
{\displaystyle g}
g
N
{\displaystyle gN}
G
→
G
/
N
{\displaystyle G\to G/N}
泛性質 的一般抽象考慮。陪集
e
N
=
N
{\displaystyle eN=N}
g
N
{\displaystyle gN}
(
g
N
)
−
1
=
g
−
1
N
{\displaystyle (gN)^{-1}=g^{-1}N}
[e]
⋅
{\displaystyle \cdot }
R
{\displaystyle R}
U
{\displaystyle U}
R
{\displaystyle R}
R
{\displaystyle R}
U
{\displaystyle U}
U
{\displaystyle U}
U
{\displaystyle U}
R
{\displaystyle R}
商群
D
4
R
{\displaystyle D_{4}R}
商群
D
4
/
R
{\displaystyle D_{4}/R}
R
{\displaystyle R}
U
=
f
v
R
{\displaystyle U=f_{v}R}
U
U
=
(
f
v
R
)
(
f
v
R
)
=
(
f
v
f
v
)
R
=
R
{\displaystyle UU=(f_{v}R)(f_{v}R)=(f_{v}f_{v})R=R}
R
=
{
id
,
r
1
,
r
2
,
r
3
}
{\displaystyle R=\left\{\operatorname {id} ,\,r_{1},\,r_{2},\,r_{3}\right\}}
D
4
/
R
{\displaystyle D_{4}/R}
交換 ,而
D
4
{\displaystyle D_{4}}
R
{\displaystyle R}
D
4
/
R
{\displaystyle D_{4}/R}
D
4
{\displaystyle D_{4}}
半直積 的概念。
商群和子群一起形成了用它的展示 描述所有群的一種方法:任何群都是這個群的生成元 上的自由群 模以“關係”子群得到的商群。例如,二面體群
D
4
{\displaystyle D_{4}}
r
{\displaystyle r}
f
{\displaystyle f}
r
=
r
1
{\displaystyle r=r_{1}}
f
=
f
v
{\displaystyle f=f_{\mathrm {v} }}
r
4
=
f
2
=
(
r
f
)
2
=
1
{\displaystyle r^{4}=f^{2}=(rf)^{2}=1}
[ 26] 關係在一起,這個群就完全描述出來了。群的表示還可以被用來構造凱萊圖 ,它是一种利用图形来辅助理解离散群 的工具。
此外,子群與商群以下列方式聯係起來:
G
{\displaystyle G}
H
{\displaystyle H}
單射
H
↣
G
{\displaystyle H\rightarrowtail G}
G
{\displaystyle G}
正規子群
N
{\displaystyle N}
G
/
N
{\displaystyle G/N}
滿射 ,即典範同態
π
:
G
↠
G
/
N
{\displaystyle \pi \colon G\twoheadrightarrow G/N}
[y]
如果同一個群中的兩個元素p 和q 滿足關係:p = x−1 qx ,其中x 也是同一個群中的元素,則稱元素p 和q 共軛 。共軛关系是一个等价关系 ,即它满足三个性质:共軛是自反 的、对称 的和傳遞 的。
在群中可以找到一個集合,這個集合中每一個元素都相互共軛,而在這個集合以外群的其他部分已經沒有任何元素與他們具有共軛關係了。稱这种集合為群中的一個共軛類 。同一個群的兩個類之間一定沒有共同的元素。群中一個元素一定屬於且僅屬於一個類。如果群中沒有元素與該元素共軛,則該元素自成一類。
群中元素個數稱為群G 的階 ,記為|G |[ 27]
子群的階能整除這個群的階[ 28]
平面減去一個(粗體)點的基本群由在這個區域內的環路構成。
群的例子和應用大量存在。起點是上面介紹過的整數的群 Z 帶有加法作為群運算。如果把加法替代為乘法 ,就得到了乘法群 。這些群是抽象代數 中重要概念的前身。
群應用於很多數學領域中。數學物件 的性质經常是通過将群關聯 与数学对象关联,并研究相應的群的性質来研究的。例如,儒勒·昂利·庞加莱 通過引入基本群 創立了現在所謂的代數拓撲 。[ 29] 拓撲性質 比如臨近 和連續 轉換成了群的性質。[i] 零同倫 (因此是無關緊要的),因為它可以收縮 為一個點。圓孔的存在防止了橙色環路被收縮。橙色環路(或任何環繞 這個圓孔一次的其他環路)所生成的,去掉了一個點的平面的基本群是無限循環群。基本群以這種方式探測到了這個圓孔。
在更新近的應用中,影響已經被倒轉過來,由群論背景來激發幾何結構了。[j] 幾何群論 采用了幾何概念,比如在雙曲群 的研究中。[ 30] 代數幾何 和數論 。例如,典型群 和皮卡德群 在代数几何上有重要应用;參見[ 31]
除了上述理論應用之外,還存在很多群的實踐應用。密碼學 依賴於抽象群論方式和從計算群論 中特別是實現于有限群上的時候所得到的算法 知識的結合。[ 32] 物理 、化學 和計算機科學 都受益於這個概念。
很多數系統,比如整數和有理數享有自然給予的群結構。在某些情況下比如對于有理數,加法和乘法運算二者都引發群結構。這種系統是叫做環 和域 的更一般的代數結構的前身。
整數Z 在加法下的群记為(Z , +),它在上面已經描述了。整數帶有用乘法 替代加法的運算,(Z , ·)不形成群。閉合、結合律和單位元公理滿足,但逆元不存在:例如,a = 2a·b = 1b = 1/2,它是有理數而非整數。因此不是所有Z 的元素都有(乘法)逆元。[k]
對乘法逆元存在的要求建议了考慮分式
a
b
{\displaystyle {\frac {a}{b}}}
整數的分式(要求b 非零)叫做有理數 。[l] Q 。對于有理數帶有乘法(Q ,·), 成為群仍有一個小障礙:因為有理數0 沒有乘法逆元(就是說沒有x 使得x ·0 = 1Q , ·)仍然不是群。
但是,所有非零有理數的集合Q \{0} = {q ∈ Q , q ≠ 0}(Q \{0},·) 。[m] a /b 的逆元是b /a ,所以逆元公理也滿足。
有理數(包括0)在加法下也形成群。同時帶有加法和乘法運算產生更復雜的結構叫做環 —如果同时除法总是可能的話(如在Q 中)就是域,它在抽象代數 中占據中心位置。群論理論因此位于這些實體的理論的底層部分。[n]
對于任何素數 p ,模算術 提供了整數模以p 的乘法群 。[ 33] p 整除的整數模 p 的同余类 ,就是說兩個數被認為是等價的如果它們的差 被p 整除。例如,如果p = 5倍數 而6和−4都等價于1。群運算給出為乘法。因此4·4 = 1 ,因為通常意义下的乘積16等價於1,而5整除16 − 1 = 15 。以上事实記為
16 ≡ 1(mod 5)。 p 的首要作用是確保了兩個都不被p 整除的整數的乘積也不被p 整除,因此指示的同馀類的集合在乘法下閉合。[o] p 的整數a ,存在一個整數b 使得
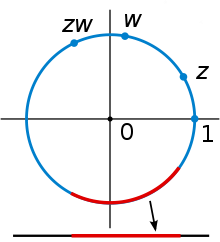
a · b ≡ 1(mod p ),就是說p 整除a ·b − 1逆元b 可以使用貝祖等式 和最大公約數 gcd(a , p ) 等于1的事實找到。[ 34] p = 53·2 = 6 ≡ 1 (mod 5) 。所有的群公理都滿足。實際上,這個例子類似于上述(Q \{0},·),因為它是在有限域F p F p × 。[ 35] 公开密钥加密 是至關重要的。[p]
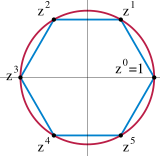
單位一的六次複數根形成一個循環群。z 是本原元而z 2 不是,因為z 的奇數冪不是z 2 的冪。 循環群 是其所有元素都是特定元素a 的冪 的群(在群運算被寫為加法的時候使用術語倍數)。[ 36]
..., a −3 , a −2 , a −1 , a 0 = e , a , a 2 , a 3 , ..., 這裡的a 2 意味著a ·a ,而a−3 表示a −1 ·a −1 ·a −1 =(a ·a ·a )−1 等等。[h] a 叫做這個群的生成元或本原元 。
這類群的典型例子是單位一的n 次複數根 ,由滿足z n 複數 z 给出,其運算為乘法。[ 37] n 個元素的循環群同構於這個群。使用某些域論 ,群F p × 可以被證明為是循環群:對于p = 5,31 = 3, 32 = 9 ≡ 4, 33 ≡ 2, 而34 ≡ 1 。無限循環群同構於(Z , +),它是前面介紹的整數在加法下的群。[ 38]
阿貝爾群包括有限生成阿貝爾群的基本定理 的研究是非常成熟的;對這個事態的反映是很多有關群論的概念,比如中心 和交換子 ,描述了一個給定群不是阿貝爾群的程度。[ 39]
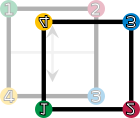
對稱群 是由給定數學對象的對稱 組成的群,對稱源于它們的幾何本性(比如前面介紹的正方形的對稱群)或源于代數本性(比如多項式 方程 和它们的解)。[ 40] [t] 數學中的對稱性 極大的簡化了幾何 或分析 對象的研究。群被稱為作用 於另一個數學對象X 上,如果所有群元素進行某個在X 上的運算兼容於群定律。在下面最右側例子中,7階的(2,3,7)三角群 的一個元素通過置換突出的彎曲的三角形作用在鑲嵌上(其他的元素也是)。通過群作用,群模式被連接到了所作用到的對象的結構上。
旋轉和翻轉形成一個大二十面體的對稱群。 在化學領域中,比如晶體學 、空間群 和點群 描述分子對稱性 和晶體對稱性。這些對稱性位于這些系統的化學和物理表現的底層,而群論使簡化對這些性質的量子力學 分析成为可能。[ 41]
群不只對評定在分子中蘊含的對稱性有用,而且令人驚奇的它們還可以預測出分子的對稱性有时候可以改变。姜-泰勒效应 是高對稱的分子的變形,此時,在通過分子的對稱運算相互關聯的一組可能基態中,该分子将采纳一個特定的低對稱的基態。[ 42] [ 43]
同樣的,群論還可以幫助預測在物質經歷相變 的時候出現的物理性質的變更,比如晶體形式從立方體變為四面體。一個例子是鐵電 物質,這裡從順電到鐵電狀態的變更出現在居里溫度 時,與從高對稱順電狀態到低對稱鐵電狀態的變更有關,并伴隨著所謂的軟聲子 模式,它是在變化時轉到零頻率的振動晶格模式。[ 44]
這種自發對稱性破缺 在基本粒子物理中找到了進一步應用,這裡它的出現与戈德斯通玻色子 的出現有关。
有限對稱群比如马蒂厄群 被用于編碼理論 中,它又用于傳輸數據的糾錯 和CD播放器 中。[ 45] 微分伽羅瓦理論 ,它刻畫有已知形式的不定積分 的函數,給出何時特定微分方程 的解有良好表現的群論判定標準。[u] 幾何 不變量理論 中研究。[ 46]
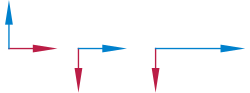
兩個向量 (左側展示),和它們乘以矩陣之后(中間和右側展示)。中間的表示了順時針旋轉90°,而右側的再按因子2伸縮了x 坐標。 矩陣群 由矩陣 加上矩陣乘法 一起構成。一般線性群GL (n , R )可逆 的n 乘n 的帶有實數 元素的矩陣構成。[ 47] 線性群 。上面提及的二面體群例子可以被看作(非常小的)矩陣群。另一個重要矩陣群是特殊正交群 SO (n )。它描述了n 維的所有可能旋轉。通過歐拉角 ,旋轉矩陣 被用于計算機圖形學 中。[ 48]
表示理論是對群概念的應用并且對深入理解群是很重要的。[ 49] [ 50] 群作用 於其他空間來研究群。一類廣泛的群表示 是線性表示,就是說群作用在線性空間 中,比如三維歐幾里得空間 R 3 。G 在n -維 實向量空間上的表示簡單的是從群到一般線性群的群同態
ρ : G → GL (n , R )。以這種方式,抽象給出的群運算被轉換成用明確的計算可觸及到的矩陣乘法。[w]
給定一個群作用,這給出了研究所作用的對象的進一步方法。[x] 代數群 和拓撲群 特別是(局部)緊群 理論中的起組織作用的原則。[ 49] [ 51]
伽羅瓦群 是通过对求解多項式 方程 的过程中涉及到的对称性的研究而被发展起来的。[ 52] [ 53] 二次方程 ax 2 + bx + c = 0
x
=
−
b
±
b
2
−
4
a
c
2
a
{\displaystyle x={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}}
對換表達式中的"+"和"−",也就是置換方程的兩個解可以被看作(非常簡單的)群運算。類似的公式對於三次方程 和四次方程 也有,但是對於五次方程 和更高次的方程就不普遍性的存在。[ 54] 可解性 )給出了那些多項式的所有解都可用根式表達的判定標準,就是說這些解可以類似上面公式那樣只使用加法、乘法和方根 來表達。[ 55]
這個問題可以使用域論 來處理:考慮一個多項式的分裂域 就把問題轉移到了域論的領域中了。現代伽羅瓦理論 把上述類型的伽羅瓦群推廣到了域擴張 ,并通過伽羅瓦理論基本定理 建立了在域和群之間的嚴格關聯,再次凸顯了群在數學中無所不在。
一個群被稱為有限群,如果它有有限 個元素。元素的數目叫做群G 的階 。[ 56] n次对称群 S N N 個字母的置換 的群。例如,在3個字母上的n次对称群S 3 ABC 的所有可能置換構成的群,就是說它包含元素ABC , ACB , ...,直到CBA ,總共有6(或3的階乘 )個元素。這類群是基礎性的,因為任何有限群都可以表達為n 次对称群S N N 下的子群(凱萊定理 )。相似於上述正方形的對稱的群,S 3 還可以解釋為等邊三角形 的對稱的群。
在群G 中的一個元素a 的階是最小的使得an = e 的正整數n ,這裡的 an 表示
a
⋅
…
⋅
a
⏟
n
{\displaystyle \underbrace {a\cdot \ldots \cdot a} _{n}}
a 的n 個復本上。(如果·代表乘法則a n a 的n 次冪)。在無限群中,這個n 可能不存在,在這種情況下a 的階被稱為無限的。一個元素的階等于這個元素生成的循環子群的階。
更復雜的計數技術例如計數陪集,產生關于有限群的更精確陳述:拉格朗日定理 聲稱有限群G 的任何有限子群H 的階整除 G 的階。西羅定理 證明了它的部分逆命題。
上面討論的二面體群 是8階有限群。r1 的階為4,這是它生成的子群R (見上)的階。反射元素fv 等的階是2。如拉格朗日定理所述這兩個階都整除8。上面的群F p × 有階p − 1
数学家们常常为寻求一种数学对象的完备的分类(或列表)而努力。并且这种分类是十分有用的: 如果有限群有一个完备的列表, 假设我们需要证明定理P 时, 如果可以一步一步证明定理对列表中给出的群成立, 那我们即可证明定理P 在有限群的领域内成立。这个目标迅速引出了一系列困难而意义深远的数学问题。拉格朗日定理 ,p阶有限群(p为素数)必定是循环(阿贝尔群 )群Z p p 2 阶群也被证明是阿贝尔群。但这一命题并不能推广到p 3 阶群,如上面的非阿贝尔群——8阶二面体群
D
4
{\displaystyle \mathrm {D} _{4}}
3 。[ 57] 计算机代数系统 来给较小的群列表 ,但没有对一切有限群的分类。[q] [r] 平凡群 仅有的正规子群是平凡群和它自身,那么这个群叫做一个单群 或简单群。[s] 合成列 说明单群可以作为建构有限群的“砖块”。[ 58] 有限单群分类 是当代群论的一个主要成就。1998年的菲尔兹奖 得主理查德·博赫兹 成功地证明了怪兽月光理论 。该猜想指出了有限单群中分类中的最大的散在群 ——“怪兽群 ”与一种来自经典复分析 和弦理论 (一种被认为统一了对许多物理学现象的描述的理论)的对象模函数 之间的惊人而深刻的联系。[ 59]
很多群同時是群和其他數學結構的例子。用范疇論 的語言來說,它們是在范疇 中的“群”物件,這意味著它們是帶著模仿群公理的(叫做態射 的)變換的對象(可以是其他代数/数学結構)。例如,所有群(如上面定義的)也是一個集合,所以群是在集合范疇 中的群物件。
在複平面 中的單位圓 在複數乘法下是李群,所以是拓撲群。它是拓撲的因為複數乘法和除法是連續的。它是流形并因此是李群,因為所有小段 比如在圖中的紅色圓弧,看起來像(顯示在底下的)實數線 的一部分。 某些拓撲空間 可以配備上群结构。為了讓群公理與拓撲交織良好,群運算必須是連續函數 ,就是說如果g 和h 只變化很小,那么g ·h ,g −1 必須變化不大。這種群叫做拓撲群,并且它們是在拓撲空間范疇 內的群對象。[ 60] 實數 R 在加法之下(R \{0},·) ,任何其他拓撲域 比如複數 或p進數 也是類似。所有這些群都是局部緊拓撲群 ,所以它們有哈尔测度 并可以通過調和分析 來研究。前者提供了不變積分 的抽象形式化。以實數情況为例,不变性意味着有:
∫
f
(
x
)
d
x
=
∫
f
(
x
+
c
)
d
x
{\displaystyle \int f(x)\,dx=\int f(x+c)\,dx}
對於任何常數c 成立。在這些域上的矩陣群也属于这种结构下,賦值向量環 和賦值向量代數群 也是如此,它們對數論是基礎性的。[ 61] 絕對伽羅瓦群 也可以配備上拓撲,叫做Krull拓撲 ,它又是推廣上面概述的域和群的連接到無限域擴張的中心概念。[ 62] 代數幾何 需要的這個想法的高級推廣是étale基本群 。[ 63]
李群(为纪念索菲斯·李 而命名)是具有流形 結構的群,就是說它們是局部上看起來像 某個適當維度 的歐幾里得空間 的空間。[ 64] 光滑 的。
標準例子是上面介紹的一般線性群:它是所有
n
×
n
{\displaystyle n\times n}
開子集 ,因為它由不等式
det (A ) ≠ 0, 給出。這裡的A 指示
n
×
n
{\displaystyle n\times n}
[ 65]
李群在物理中是基礎性的:诺特定理 把連續對稱与守恒定律 关联起来。[ 66] 空間 和時間 中旋轉 和平移 不变性是力學 定律的基本對稱。它們可以被用來構造簡單的模型——比如在一種狀況下實施軸對稱常常會導致在解用來提供物理描述的方程上的重大簡化。[v] 洛伦兹变换 ,它有關於兩個相互運動的觀察者的時間和速度的測量。它們可以用純群論方式推演,通過把變換表達為闵可夫斯基時空 的旋轉對稱。在忽略萬有引力 的情況下,后者充當了狹義相對論 的時空 模型。[ 67] 庞加莱群 。通過上述联系,它在狹義相對論中扮演了關鍵角色,并隐含地用于量子場論 。[ 68] 隨位置變化的對稱 与規范場論 一起构成现代物理对相互作用的描述的中心。[ 69]
在抽象代數 中,通過放松定義群的某個公理可定義出更多的一般結構。[ 21] [ 70] [ 71] 幺半群 。自然數 集N (包括0)在加法下形成了幺半群,還有非零整數在乘法下(Z \{0},·) 也是。有一種一般方法用來向任何(阿貝爾的)幺半群正式的增加元素的逆元,非常類似于從(Z \{0},·) 得出(Q \{0},·) 的方式,這叫做格罗滕迪克群 。广群 非常類似于群,除了復合a · b 不必須在所有的a 和b 上有定義之外。它們由更加復雜形式的對稱的研究所引發,常見于拓撲 和分析 結構比如基本广群 中。表格給出一些推廣群的結構。
^ a: 《
數學評論 》列出了3,224篇2005年寫的關于群論和它的應用的研究論文。
^ b: 閉合公理已經由·是二元運算的條件所蘊含。因此有些作者省略這個公理。
Lang 2002 。
^ c: 比如參見Lang (2002, 2005)和Herstein (1996, 1975)的書。
^ d: 但是一個群不由它的子群的格所決定。參見
Suzuki 1951 。
^ f: 例如,依據拉格朗日定理,如果
G 是有限的,則任何子群和任何商群的
大小 整除
G 的大小。
^ h: 循環群的加法符號是
t ·a , t ∈ Z 。
^ k: 有乘法逆元的元素叫做
可逆元 ,參見
Lang 2002 , §II.1, p. 84 。
^ l: 通過增加分數的從整數到有理數的轉變推廣為
分式域 。
^ m: 用任何
域 F 替代
Q 同樣是真的。參見
Lang 2005 , §III.1, p. 86 。
^ n: 例如,域的乘法群的有限子群必然是循環群。參見
Lang 2002 , Theorem IV.1.9 。
模 和
單純代數 的
撓 概念是這個原理的另一個實例。
^ o: 陳述的性質是素數的一個可能定義。參見
素元 。
^ q: 阶不超过2000的群是已知的。这些群在同构意义下约有490亿个。参见
Besche, Eick & O'Brien 2001 .
^ r: 在单群和一般群分类之间的缺口在于
扩张问题 ,一个很难一般性求解的问题。参见
Aschbacher 2004 , p. 737 .
^ s: 等价地说,一个非平凡群是单群当且仅当它仅有的商群是平凡群和自身。参见
Michler 2006 ,
Carter 1989 .
^ t: 更嚴格的說,所有群都是某個
圖 的對稱群,參見
Frucht 1939 。
^ v: 例如參見
史瓦西度规 ,這裡的對稱極大的減小了物理系統的復雜性。
^ w: 例如,這是有限簡單群的分類的關鍵。參見
Aschbacher 2004 。
^ Herstein 1975 , §2, p. 26 ^ Hall 1967 , §1.1, p. 1 :“群的想法遍布在包括純數學和應用數學二者的整個數學中。”^ Wussing 2007 ^ Kleiner 1986 ^ Smith 1906 ^ Galois 1908 ^ Kleiner 1986 , p. 202 ^ Cayley 1889 ^ Wussing 2007 , §III.2 ^ Lie 1973 ^ Kleiner 1986 , p. 204 ^ Wussing 2007 , §I.3.4 ^ Jordan 1870 ^ von Dyck 1882 ^ Curtis 2003 ^ Mackey 1976 ^ Borel 2001 ^ Aschbacher 2004 ^ Herstein 1975 , §2.1, p. 27 ^ Lang 2005 , App. 2, p. 360 ^ 21.0 21.1 Mac Lane 1998 ^ Lang 2005 , §II.3, p. 34 ^ Lang 2005 , §II.1, p. 19 ^ Ledermann 1973 , §II.12, p. 39 ^ Lang 2005 , §II.4, p. 41 ^ Lang 2002 , §I.2, p. 9 ^ 韩士安,林磊. 近世代数(第二版). 北京: 科学出版社. 2009: 8. ISBN 9787030250612 ^ [美] 约翰·德比希尔 . 代数的历史:人类对未知量的不舍追踪(修订版). 人民邮电出版社. ISBN 9787115225375(简体中文) . 关于群结构的第一个伟大定理是拉格朗日定理:子群的阶整除这个群的阶。整除的商被称为这个子群的指数。根据拉格朗日定理,分数指数不会出现。我们可以在 6 阶群中找到阶为 2 或 3(指数分别为 3 或 2)的子群,但是我们永远不可能在其中找到阶为 4 或 5 的子群,因为 6 不能被 4 或 5 整除。 ^ Hatcher 2002 , Chapter I, p. 30 ^ Coornaert, Delzant & Papadopoulos 1990 ^ Neukirch 1999 ,特別是§§I.12和I.13^ Seress 1997 ^ Lang 2005 , Chapter VII ^ Rosen 2000 , p. 54 (Theorem 2.1) ^ Lang 2005 , §VIII.1, p. 292 ^ Lang 2005 , §II.1, p. 22 ^ Lang 2005 , §II.2, p. 26 ^ Lang 2005 , §II.1, p. 22 (example 11) ^ Lang 2002 , §I.5, p. 26, 29 ^ Weyl 1952 ^ Conway, Delgado Friedrichs & Huson et al. 2001 .另见Bishop 1993 ^ Bersuker, Isaac, The Jahn-Teller Effect, Cambridge University Press: 2, 2006, ISBN 0521822122 ^ Jahn & Teller 1937 ^ Dove, Martin T, Structure and Dynamics: an atomic view of materials, Oxford University Press: 265, 2003, ISBN 0198506783 ^ Welsh 1989 ^ Mumford, Fogarty & Kirwan 1994 ^ Lay 2003 ^ Kuipers 1999 ^ 49.0 49.1 Fulton & Harris 1991 ^ Serre 1977 ^ Rudin 1990 ^ Robinson 1996 , p. viii ^ Artin 1998 ^ Lang 2002 , Chapter VI (see in particular p. 273 for concrete examples)^ Lang 2002 , p. 292 (Theorem VI.7.2) ^ Kurzweil & Stellmacher 2004 ^ Artin 1991 , Theorem 6.1.14 .另见Lang 2002 , p. 77 ,其中包含类似结果。^ Lang 2002 , §I. 3, p. 22 ^ Ronan 2007 ^ Husain 1966 ^ Neukirch 1999 ^ Shatz 1972 ^ Milne 1980 ^ Warner 1983 ^ Borel 1991 ^ Goldstein 1980 ^ Weinberg 1972 ^ Naber 2003 ^ Becchi 1997 ^ Denecke & Wismath 2002 ^ Romanowska & Smith 2002
Artin, Michael, Algebra, Prentice Hall, 1991, ISBN 978-0-89871-510-1 Devlin, Keith, The Language of Mathematics: Making the Invisible Visible, Owl Books, 2000, ISBN 978-0-8050-7254-9 Fulton, William; Harris, Joe, Representation theory. A first course, Graduate Texts in Mathematics , Readings in Mathematics 129 , New York: Springer-Verlag, 1991, ISBN 978-0-387-97495-8MR 1153249 , ISBN 978-0-387-97527-6 Hall, G. G., Applied group theory, American Elsevier Publishing Co., Inc., New York, 1967, MR 0219593 Herstein, Israel Nathan, Abstract algebra 3rd, Upper Saddle River, NJ: Prentice Hall Inc., 1996, ISBN 978-0-13-374562-7MR 1375019 Herstein, Israel Nathan, Topics in algebra 2nd, Lexington, Mass.: Xerox College Publishing, 1975, MR 0356988 Lang, Serge , Algebra, Graduate Texts in Mathematics 211 , Berlin, New York, 2002, ISBN 978-0-387-95385-4MR 1878556 Lang, Serge, Undergraduate Algebra 3rd, Berlin, New York: Springer-Verlag, 2005, ISBN 978-0-387-22025-3 Ledermann, Walter, Introduction to the theory of finite groups, Oliver and Boyd, Edinburgh and London, 1953, MR 0054593 Ledermann, Walter, Introduction to group theory, New York: Barnes and Noble, 1973, OCLC 795613 Robinson, Derek John Scott, A course in the theory of groups, Berlin, New York: Springer-Verlag, 1996, ISBN 978-0-387-94461-6 《代數學引論》第二版ISBN 7-04-008893-2聶靈沼、丁石孫著,高等教育出版社 出版
Artin, Emil , Galois Theory, New York: Dover Publications, 1998, ISBN 978-0-486-62342-9 Aschbacher, Michael, The Status of the Classification of the Finite Simple Groups (PDF) , Notices of the American Mathematical Society, 2004, 51 (7): 736–740 [2008-09-13 ] , ISSN 0002-9920 存档 (PDF) 于2011-06-05) Becchi, C., Introduction to Gauge Theories , 1997 [2008-05-15 ] , (原始内容存档 于2016-10-07) Besche, Hans Ulrich; Eick, Bettina; O'Brien, E. A., The groups of order at most 2000 , Electronic Research Announcements of the American Mathematical Society, 2001, 7 : 1–4 [2008-09-13 ] , doi:10.1090/S1079-6762-01-00087-7 MR 1826989 , (原始内容存档 于2008-10-08) Bishop, David H. L., Group theory and chemistry, New York: Dover Publications, 1993, ISBN 978-0-486-67355-4 Borel, Armand, Linear algebraic groups, Graduate Texts in Mathematics 126 2nd, Berlin, New York: Springer-Verlag, 1991, ISBN 978-0-387-97370-8MR 1102012 Carter, Roger W., Simple groups of Lie type, New York: John Wiley & Sons, 1989, ISBN 978-0-471-50683-6 Conway, John Horton ; Delgado Friedrichs, Olaf; Huson, Daniel H.; Thurston, William P. , On three-dimensional space groups , Beiträge zur Algebra und Geometrie, 2001, 42 (2): 475–507 [2008-09-13 ] , ISSN 0138-4821 MR 1865535 , (原始内容存档 于2020-06-01) (法文) Coornaert, M.; Delzant, T.; Papadopoulos, A., Géométrie et théorie des groupes [Geometry and Group Theory], Lecture Notes in Mathematics 1441 , Berlin, New York: Springer-Verlag, 1990, ISBN 978-3-540-52977-4MR 1075994 Denecke, Klaus; Wismath, Shelly L., Universal algebra and applications in theoretical computer science, London: CRC Press, 2002, ISBN 978-1-58488-254-1 Fröhlich, Albrecht, Formal groups, Lecture notes in mathematics 74 , Berlin, New York: Springer-Verlag, 1968 (德文) Frucht, R., Herstellung von Graphen mit vorgegebener abstrakter Gruppe [Construction of Graphs with Prescribed Group] , Compositio Mathematica, 1939, 6 : 239–50, ISSN 0010-437X 原始内容 存档于2008-12-01) Goldstein, Herbert, Classical Mechanics 2nd, Reading, MA: Addison-Wesley Publishing: 588–596, 1980, ISBN 0-201-02918-9 Hatcher, Allen, Algebraic topology , Cambridge University Press , 2002 [2008-09-13 ] , ISBN 978-0-521-79540-1存档 于2018-05-19) Husain, Taqdir, Introduction to Topological Groups, Philadelphia: W.B. Saunders Company, 1966, ISBN 978-0-89874-193-3 Jahn, H.; Teller, E. , Stability of Polyatomic Molecules in Degenerate Electronic States. I. Orbital Degeneracy, Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences (1934-1990), 1937, 161 (905): 220–235, doi:10.1098/rspa.1937.0142 Kassel, Christian, Quantum Groups, Springer, 1994, ISBN 978-0387943701 Kuipers, Jack B., Quaternions and rotation sequences - A primer with applications to orbits, aerospace, and virtual reality, Princeton University Press, 1999, ISBN 978-0-691-05872-6MR 1670862 Kuga, Michio, Galois' dream: group theory and differential equations, Boston, MA: Birkhäuser Boston, 1993, ISBN 978-0-8176-3688-3MR 1199112 Kurzweil, Hans; Stellmacher, Bernd, The theory of finite groups, Universitext, Berlin, New York: Springer-Verlag, 2004, ISBN 978-0-387-40510-0MR 2014408 Lay, David, Linear Algebra and Its Applications, Addison-Wesley, 2003, ISBN 978-0-201-70970-4 Mac Lane, Saunders, Categories for the Working Mathematician 2nd, Berlin, New York: Springer-Verlag, 1998, ISBN 978-0-387-98403-2 Michler, Gerhard, Theory of finite simple groups, Cambridge University Press , 2006, ISBN 978-0-521-86625-5 Milne, James S., Étale cohomology, Princeton University Press, 1980, ISBN 978-0-691-08238-7 Mumford, David ; Fogarty, J.; Kirwan, F., Geometric invariant theory 34 3rd, Berlin, New York: Springer-Verlag, 1994, ISBN 978-3-540-56963-3MR 1304906 Naber, Gregory L., The geometry of Minkowski spacetime, New York: Dover Publications, 2003, ISBN 978-0-486-43235-9MR 2044239 Neukirch, Jürgen, Algebraic Number Theory, Grundlehren der mathematischen Wissenschaften 322 , Berlin: Springer-Verlag, 1999, ISBN 978-3-540-65399-8MR 1697859 Romanowska, A.B.; Smith, J.D.H., Modes, World Scientific, 2002, ISBN 9789810249427 Ronan, Mark, Symmetry and the Monster: The Story of One of the Greatest Quests of Mathematics, Oxford University Press, 2007, ISBN 978-0-19-280723-6 Rosen, Kenneth H., Elementary number theory and its applications 4th, Addison-Wesley, 2000, ISBN 978-0-201-87073-2MR 1739433 Rudin, Walter, Fourier Analysis on Groups, Wiley Classics, Wiley-Blackwell, 1990, ISBN 047152364X Seress, Ákos, An introduction to computational group theory , Notices of the American Mathematical Society, 1997, 44 (6): 671–679, ISSN 0002-9920 MR 1452069 , (原始内容 存档于2007-02-08) Serre, Jean-Pierre , Linear representations of finite groups, Berlin, New York: Springer-Verlag, 1977, ISBN 978-0-387-90190-9MR 0450380 Shatz, Stephen S., Profinite groups, arithmetic, and geometry, Princeton University Press, 1972, ISBN 978-0-691-08017-8MR 0347778 Suzuki, Michio, On the lattice of subgroups of finite groups, Transactions of the American Mathematical Society, 1951, 70 (2): 345–371, doi:10.2307/1990375 Warner, Frank, Foundations of Differentiable Manifolds and Lie Groups, Berlin, New York: Springer-Verlag, 1983, ISBN 978-0-387-90894-6 Weinberg, Steven , Gravitation and Cosmology, New York: John Wiley & Sons, 1972, ISBN 0-471-92567-5 Welsh, Dominic, Codes and cryptography, Oxford: Clarendon Press, 1989, ISBN 978-0-19-853287-3 Weyl, Hermann, Symmetry, Princeton University Press, 1952, ISBN 978-0-691-02374-8
Borel, Armand, Essays in the History of Lie Groups and Algebraic Groups, Providence, R.I.: American Mathematical Society , 2001, ISBN 978-0-8218-0288-5 Cayley, Arthur , The collected mathematical papers of Arthur Cayley , II (1851 - 1860), Cambridge University Press , 1889 Curtis, Charles W., Pioneers of Representation Theory: Frobenius, Burnside, Schur, and Brauer, History of Mathematics, Providence, R.I.: American Mathematical Society , 2003, ISBN 978-0-8218-2677-5 (德文) von Dyck, Walther, Gruppentheoretische Studien Group-theoretical Studies (subscription required) , Mathematische Annalen, 1882, 20 (1): 1–44, ISSN 0025-5831 doi:10.1007/BF01443322 [永久失效連結 (法文) Galois, Évariste , Tannery, Jules , 编, Manuscrits de Évariste Galois [Évariste Galois' Manuscripts] , Paris: Gauthier-Villars, 1908 [2008-09-13 ] , (原始内容存档 于2011-05-21) Joseph Liouville in 1843).(法文) Jordan, Camille, Traité des substitutions et des équations algébriques [Study of Substitutions and Algebraic Equations] , Paris: Gauthier-Villars, 1870 Kleiner, Israel, The evolution of group theory: a brief survey (subscription required) , Mathematics Magazine, 1986, 59 (4): 195–215, ISSN 0025-570X MR 863090 (德文) Lie, Sophus , Gesammelte Abhandlungen. Band 1 [Collected papers. Volume 1], New York: Johnson Reprint Corp., 1973, MR 0392459 Mackey, George Whitelaw, The theory of unitary group representations, University of Chicago Press, 1976, MR 0396826 Smith, David Eugene, History of Modern Mathematics , Mathematical Monographs, No. 1, 1906 [2008-09-13 ] , (原始内容存档 于2008-09-19) Wussing, Hans, The Genesis of the Abstract Group Concept: A Contribution to the History of the Origin of Abstract Group Theory, New York: Dover Publications, 2007, ISBN 978-0-486-45868-7





























![{\displaystyle {\begin{aligned}e_{l}&=\Gamma \circ (g\circ \gamma _{l})\\&=\Gamma \circ [\,g\circ (e_{l}\circ \gamma _{l})\,]\\&=\Gamma \circ \{\,g\circ [\,(\gamma _{l}\circ g)\circ \gamma _{l}\,]\,\}\\&=\Gamma \circ \{\,g\circ [\,\gamma _{l}\circ (g\circ \gamma _{l})\,]\,\}\\&=\Gamma \circ [\,(g\circ \gamma _{l})\circ (g\circ \gamma _{l})\,]\\&=[\,\Gamma \circ (g\circ \gamma _{l})\,]\circ (g\circ \gamma _{l})\\&=e_{l}\circ (g\circ \gamma _{l})\\&=g\circ \gamma _{l}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9260ce7ca76a68fceab377473184f8bb95e74ac0)








![{\displaystyle {\begin{aligned}e_{r}&=(\gamma _{r}\circ g)\circ {\overline {\Gamma }}\\&=[\,(\gamma _{r}\circ e_{r})\circ g\,]\circ {\overline {\Gamma }}\\&=\{\,[\,\gamma _{r}\circ (g\circ \gamma _{r})\,]\circ g\,\}\circ {\overline {\Gamma }}\\&=\{\,[\,(\gamma _{r}\circ g)\circ \gamma _{r}\,]\circ g\,\}\circ {\overline {\Gamma }}\\&=[\,(\gamma _{r}\circ g)\circ (\gamma _{r}\circ g)\,]\circ {\overline {\Gamma }}\\&=(\gamma _{r}\circ g)\circ [\,(\gamma _{r}\circ g)\circ {\overline {\Gamma }}\,]\\&=(\gamma _{r}\circ g)\circ e_{r}\\&=\gamma _{r}\circ g\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a84c136a0a36ffce47eebbf8629dede6b997a030)
















































![{\displaystyle [\neg {\mathcal {B}}\wedge (e_{(G,\,\circ )}=\varnothing )]\vee [{\mathcal {B}}\wedge (\forall g\in G)(e_{(G,\,\circ )}\circ g=g\circ e_{(G,\,\circ )}=g)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/326e948a2c24cdf9e3dca413b84cbb99da36f4b7)







![{\displaystyle [\neg {\mathcal {D}}\wedge ({g^{-1}}_{(G,\,\circ )}=\varnothing )]\vee [{\mathcal {D}}\wedge ({g^{-1}}_{(G,\,\circ )}\circ g={g^{-1}}_{(G,\,\circ )}\circ g=e_{G})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4e13b0b17cf9fd36a248497554f1a15f0823ab35)































![{\displaystyle \forall a\in G,\,\varphi (a^{-1})=\left[\varphi (a)\right]^{-1}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/894ae41cb651386501dcc15814802c9f0b0f206c)












































































